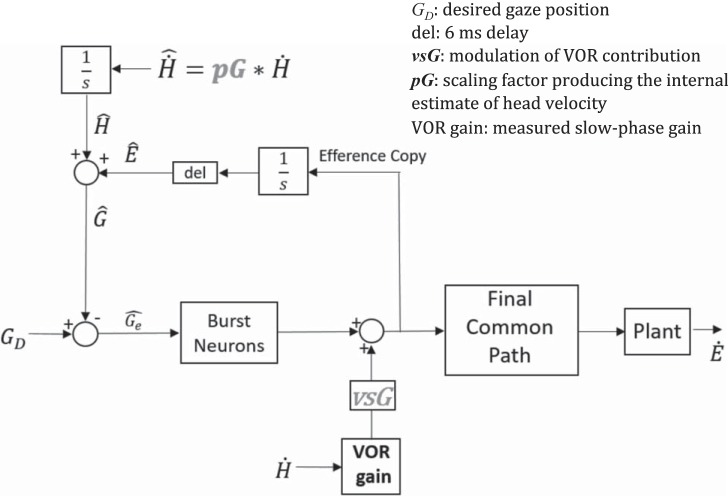
Fig. 2.

Mathematical modeling of gaze correction: the brain computes an estimate of head velocity ($\widehat{\stackrel{․}{H}}$) relying on multisensory information. We modeled $\widehat{\stackrel{․}{H}}$ with a gain (pG) that multiplies actual head velocity. An estimate of gaze position $\widehat{G}$ is thus computed by adding an estimate of eye position $\widehat{E}$ provided by the resettable integrator in the local feedback loop to the estimate of head position, $\widehat{H}$. This estimate is compared with the desired gaze ($G_D$ = 0 in a gaze-stabilization task) and their difference, gaze error (${\widehat{G}}_{\mathrm{e}}$), drives saccadic burst neurons that move the eyes until $\widehat{G}=G_D$, when the saccade stops. The VOR signal is multiplied by a coefficient (vsG), ranging between 0 and 1: if vsG = 1, the (deficient) input from the labyrinth is added to the saccadic command and sent to the final common path (linear summation hypothesis), otherwise its contribution is attenuated (0 ≤ vsG ≤ 1) or canceled (vsG = 0).