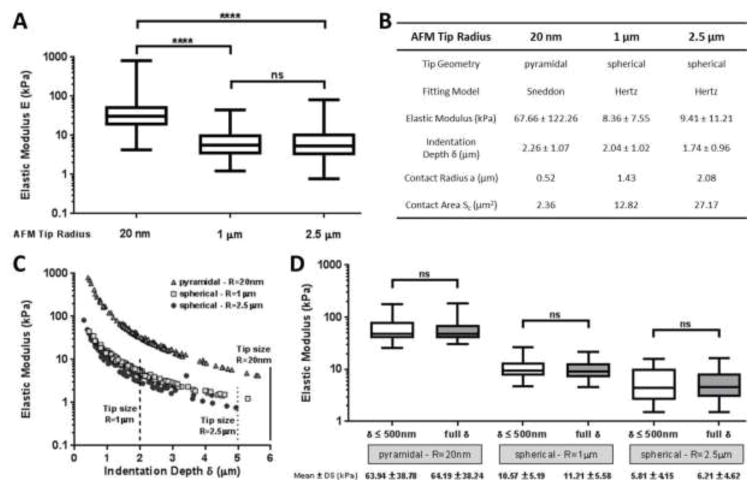
Figure 2.

(A) Elastic modulus of human pulmonary vessels estimated by AFM indentation performed with three different AFM tips: sharp pyramidal tip at 20 nm radius (half-open angle of 18°), spherical tip at 1 μm radius and spherical tip with a radius of 2.5 μm. Force curves were performed on 4 vessels from 4 different lung donors (one vessel per donor). (B) Mean ± SD of elastic modulus determined by fitting force curves with Sneddon’s (for pyramidal AFM tip) and Hertz’s (for spherical AFM tip) models. Contact radii a and areas S_c were calculated from the equations (4) and (5) for spherical tips and (7) and (8) for pyramidal tip with the mean value of the indentation depth δ. (C) Elastic modulus extracted from force curves performed with three different AFM tips on human pulmonary arteries, plotted as a function of the indentation depth. The size of the AFM tips is indicated to compare with the indentation depth values range. For the pyramidal tip, the tip height is estimated as 6 μm. (D) Elastic modulus estimated by fitting force curves considering large (entire curve) or limited (δ ≤ 500 nm) indentation depths. Analysis shown for all curves from one sample (from Table 2). Comparison of mean values for each tip was conducted using a Mann-Whitney test for each of the three AFM tips.