Figure 10.
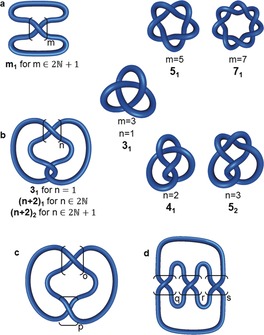
Generation patterns for torus, twist, clasp, and pretzel knots. a) For odd values of m, a torus knot X 1 is obtained (X is the number of crossings). These knots are generated by twisting two strands around each other. For even values of m, two component links are created. b) This construct gives a twist knot for any positive n. Twist knots with an even number of crossings are denoted as X 1, those with an odd number as X 2 (in this case X1 is a torus knot). c) Twist knots are a type of clasp knot C(p,o). Twist knots are C(2,o) clasp knots. d) The generation pattern for (q,r,s) pretzel knots. Pretzel knots consist of left or right‐handed helices connected together (see Section 2.11).
