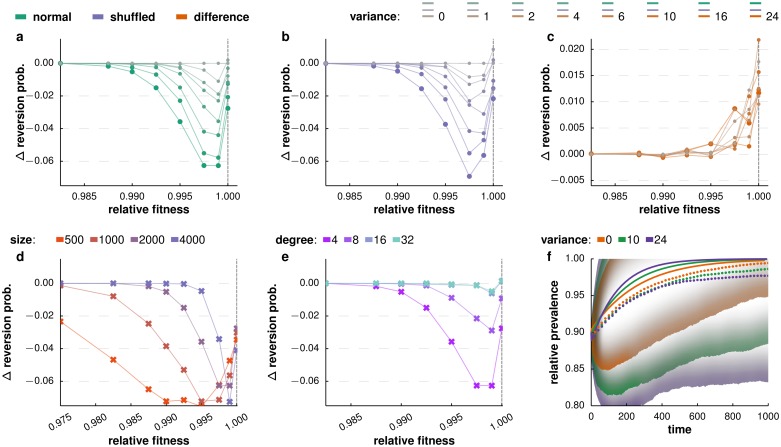
Fig 3. Characterization of the effect of degree variance on reversion probability.
a-c. The magnitude of the effect of variance in degree of the network relative to zero variance (gray lines) as a function of the relative fitness of the resistant strain, for a fraction of resistant infecteds of fr = 0.1 at a gradual treatment halt. Panel a illustrates the impact of degree variance on the probability of reversion. For values of the relative fitness of the resistant type close to but slightly smaller than 1, an increase in degree variance leads to a substantially lower probability of reversion. Panel b illustrates the effect of network occupancy. It reports the magnitude in effect of the variance in degree on the reversion probability in case of a shuffled distribution of the infection type (resistant versus wild type) among all infecteds at the end of treatment. To assess the effect of network occupancy within the infecteds at the end of treatment, panel c shows the difference between treatment halt without and treatment halt with shuffling of the infection type. We see that network occupancy has a slightly positive effect on the probability of reversion. d. The effect of variance (σ2 = 24) in degree relative to zero variance as a function of the relative fitness of the resistant strain, for a range of host population sizes. e. Impact of host network density on the relative effect of variance (σ2 = 24) as a function of the relative fitness of the resistant strain. f. Relative prevalence of the wild-type strain during the post treatment phase for a relative fitness of the mutant strain of sA = 0.995. Solid lines show the analytical solution of a two-strain pair approximation. The mean relative prevalence (dotted lines) is lower for network with a higher degree variance. The standard deviation of the mean relative prevalence (outlined with according color gradient) increases with the degree variance, indicating an increase in the magnitude of stochastic noise with increasing variance in degree of the host network.