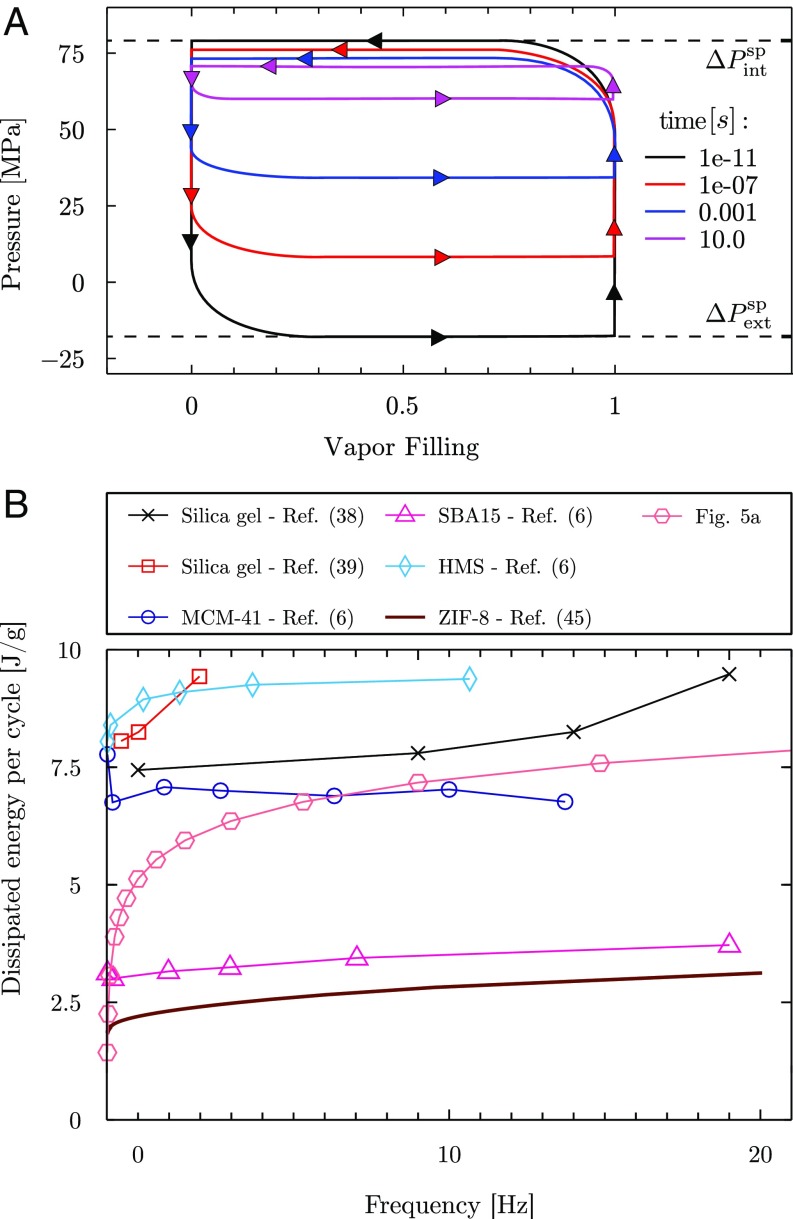
Fig. 5.

(A) Hysteresis cycle for a thought intrusion/extrusion experiment on the $D=\hspace{0.17em}2.6$-nm pore; the pressure is varied between the intrusion value $\mathrm{\Delta }{P}_{\mathrm{int}}^{\mathrm{sp}}$ and the extrusion one, $\mathrm{\Delta }{P}_{\mathrm{ext}}^{\mathrm{sp}}$ (black lines). The plot is constructed by adding a term $\mathrm{\Delta }P{V}_v$ to the atomistic free-energy profile at $\mathrm{\Delta }P=\hspace{0.17em}0$ in Fig. 4 and by computing the spinodal pressures; the compressibility of the liquid and of the pore walls is not taken into account, resulting in a rectangular cycle (only the values of the pressure at the plateaus are actually computed; rounded angles help visualization). On the $x$ axis, the bubble volume $V_v$ is normalized with the maximum value such that it ranges from $0$ (fully wet pore) to $1$ (vapor bubble occupying the nanopore). The pressures at which intrusion (top plateau) and extrusion (lower plateau) happen for fixed experimental times $t$ are computed inverting Eq. 1 to obtain the intrusion and extrusion barriers $\mathrm{\Delta }{\mathrm{\Omega }}^{†}(\mathrm{\Delta }P)$ corresponding to the prescribed $t$ (colored lines). (B) Energy $E_d$ dissipated per cycle as a function of the frequency, from refs. 6, 38, 39, and 45 and from A. Experimental data, which are available over a limited range of frequencies, were originally given in different units, which required estimates of the porosity (38, 39) or interpolation of $\mathrm{\Delta }{P}_{\mathrm{int}}$ and $\mathrm{\Delta }{P}_{\mathrm{ext}}$ (45). We remark that experiments are performed on different materials, temperatures, and, for some of them (38), $E_d$ refers to the entire device.