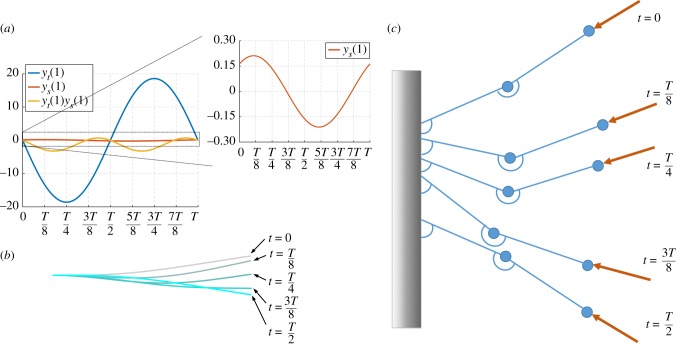
Figure 7.

Non-variational aspects of flapping motion. (a) Time evolution of the tip velocity, y_t(1), its derivative with respect to the arclength, y_s(1) (zoomed in the inset) and their product, y_t(1)y_s(1) over the period of oscillation T for σ = 37.8. The term y_t(1)y_s(1) changes sign four times over a cycle: the presence of the follower force both removes and injects energy into the system, giving rise to self-sustained, periodic oscillations. (b) Filament configuration over a half-period. (c) Schematic of the two-link model at different times over a half-period for Σ = 3.5. The follower force compresses the two-link structure and the links are pushed downwards (0 < t < T/4). Then, the first link reaches its lowest point (i.e. highest restorative moment) and stops moving, while the second link keeps rotating (t = 3T/8). By doing so, the follower force, which has followed the second link, exerts a lower moment and the restorative effect becomes predominant. Hence, the first link moves upwards and the second link downwards until stopping and inverting its motion (t = T/2). The cycle then repeats.