Figure 7.

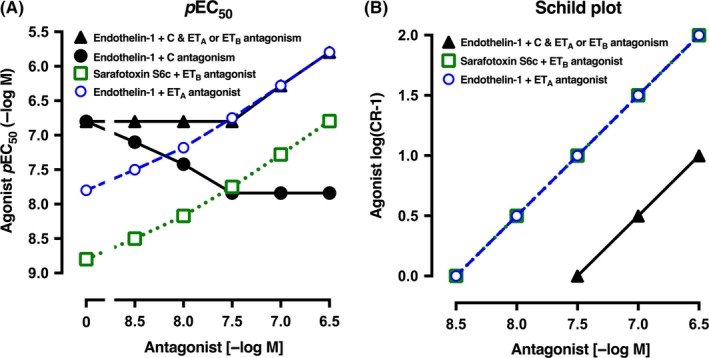
(A) Hypothetical relationship between the pEC ₅₀ values for endothelin‐1 or sarafotoxin S6c concentration‐contraction curves and the concentration of a theoretical dual ET_A and ET_B receptor antagonist with a pK_B of 8.5 at both receptors. For simplicity, the control (0 antagonist) pEC ₅₀ for sarafotoxin S6c () was set 1 log unit higher (10‐fold more potent) than for endothelin‐1 (). In the presence of ET_B receptors and the clearance (C) mechanism for endothelin‐1, the maximum clearance was set at 10‐fold (1 log unit) so that the pEC ₅₀ in the presence of no antagonist (0) rises 1 log unit (● or ▲). As the ET_B antagonism starts to block the endothelin‐1 clearance, so the pEC ₅₀ rises (●) but just as does the ET_B and ET_A antagonism so that the resultant shows the actual pEC ₅₀ is not altered. (B) The Schild plot for endothelin‐1 (or sarafotoxin S6c) as the agonist and the dual ET_A and ET_B antagonist with pK_B of 8.5 is shown. Separate theoretical lines are shown for ET_A (; eg, rat aorta) and ET_B (; eg, trachea). In the presence of ET_B‐mediated clearance (C) that removes endothelin‐1, as in pulmonary artery, the Schild plot points (▲) move parallel 1 log unit to decrease the potency of the dual antagonist by 10‐fold (ie, the pK_B of 8.5 becomes 7.5). The y axis is the agonist log(concentration ratio–1) and the x axis shows the concentration of dual ET_A and ET_B antagonist (−log M)