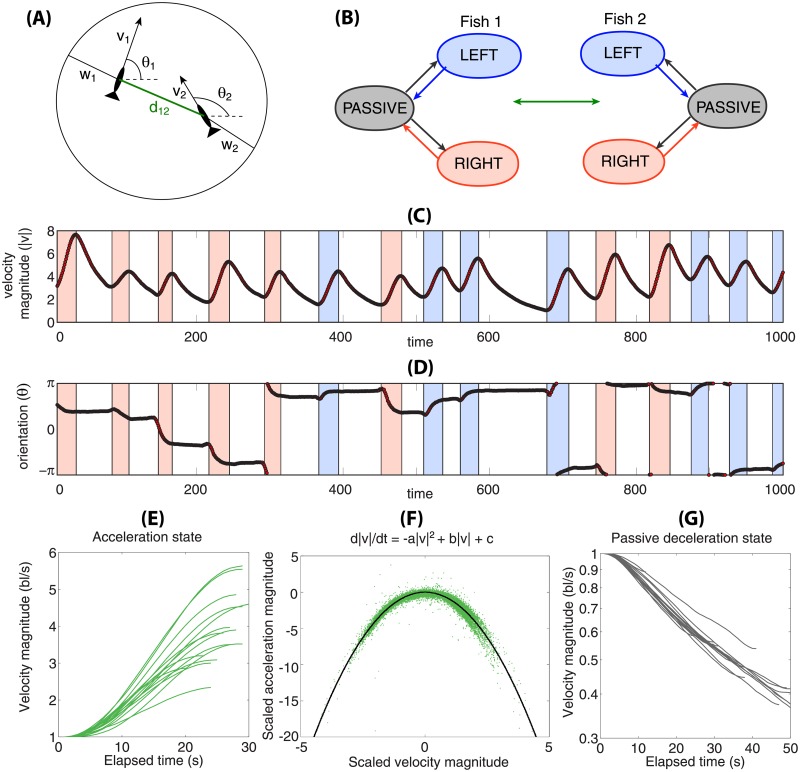
Fig 7. Motion of two fish in a circular shallow water tank.

(A) Each fish is characterized by a position (x, y), velocity v, orientation θ and acceleration a. (B) Diagram of behavioral state transitions for the two fish with interaction. (C-D) A time window of length 1000 s shows the velocity and orientation of one fish (second fish not shown) from the data in [12]. The trajectory shows alternating regions of acceleration (blue = turning left, red = turning right) or deceleration (no shade), consistent with the states marked in B. (E) Velocity traces in the accelerating phase (data from C), shifted to the same initial value, have a sigmoidal functional form. Acceleration can be fitted empirically to a quadratic function, d|v|/dt = −a|v|² + b|v| + c where $\left|v\right|=\sqrt{{\left(\dot{x}\right)}^2+{\left(\dot{y}\right)}^2}$. This is shown in (F) for data containing 3000 accelerating intervals. Data from each accelerating window (dotted green) is fitted separately and then shifted and rescaled to a normal form |a| = −|v|² (black). (G) Passive state shows an exponential decay of the velocity due to friction, evident by rescaling decelerating trajectories to the same initial value and plotting them on a log-linear scale.