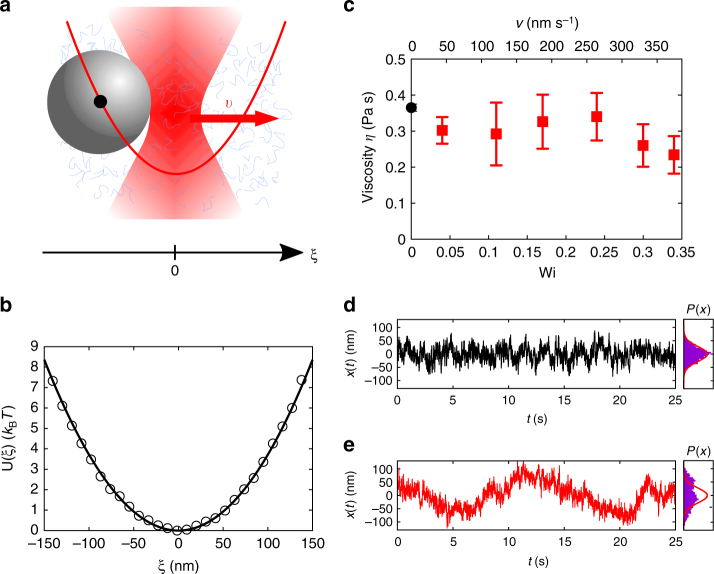
Fig. 1.

Colloidal particle in a harmonic trap driven through a viscoelastic fluid. a Micrometre-sized particle in a micellar solution and subjected to a harmonic potential, which moves with constant velocity v. b Measured trap potential (symbols) and a parabolic fit (solid line), from which the trap stiffness κ is extracted. c Viscosity η obtained from the drag force acting on the particle as a function of the trap velocity and the corresponding Weissenberg number, respectively. The value at Wi = 0 is obtained from the mean squared displacement of the particle in the absence of the trap. η is independent of the particle velocity, indicating the linear response regime. In contrast to Newtonian liquids, we observe strong differences in the fluctuations and their probability distributions P(x), i.e., for Wi = 0 (d) and for finite Wi (e). Red lines give the Boltzmann distribution in equilibrium