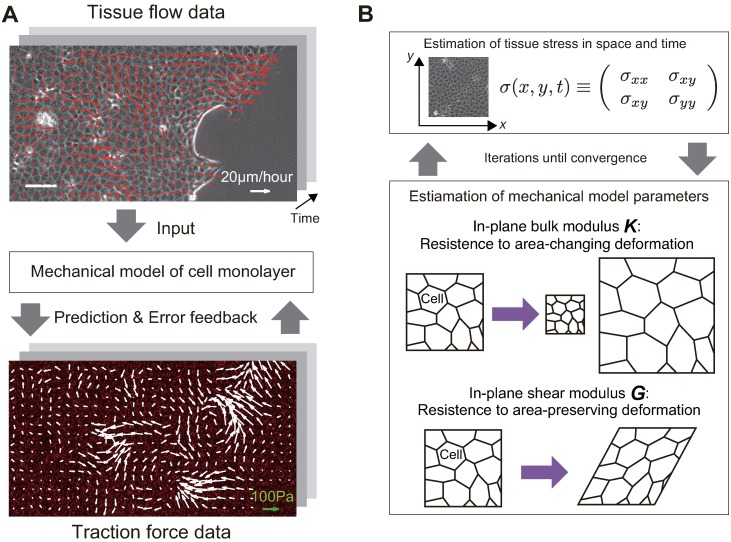
Fig 1. Overview of the inference algorithm.

(A) A relation between our model and the obtained data. In a migrating cell monolayer, cells exert traction forces on the underlying substrate, in order to propel themselves forward. Since the cells adhere to the neighboring cells, the traction force introduces mechanical stress to the tissue and induces tissue flow. We simultaneously observed the velocity field in the tissue by the phase contrast imaging (top), and the traction force by measuring the displacement of fluorescent beads embedded into the soft substrate (bottom). The continuum-mechanical model, Eqs 4 and 5, quantitatively relates the tissue flow to the traction force by considering stress as the intermediate variable. At each time point, the model describes the stress evolution under the flow, and thus predicts the traction force at the next time step. Following this, the model receives the error feedback based on the difference between predicted and observed traction forces, so that the model state (the stress) is calibrated. (B) Schematic representation of the inference algorithm. Based on the procedure outlined in (A), the algorithm estimates the tissue stress tensor in space and time. Afterward, the model parameters are updated to reproduce the estimated stress dynamics. The procedure is repeated until the convergence.