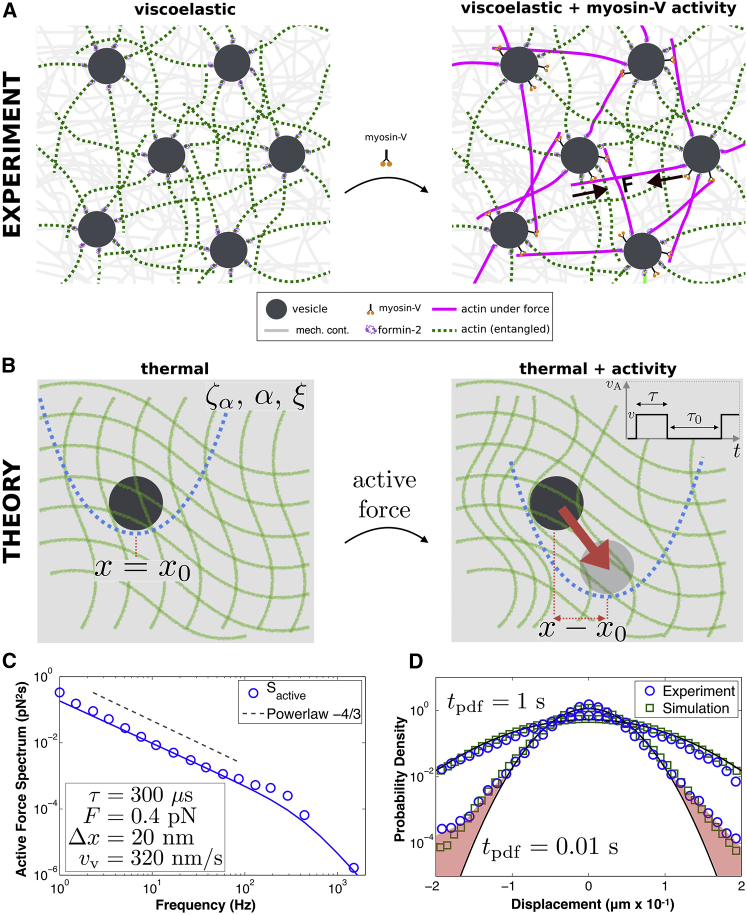
Figure 4.

A theoretical model of active mechanics connects in vivo measurements to molecular force kinetics. (A) Vesicles (dark gray) are embedded in the complex mechanical continuum (gray background) of the oocyte interior. Actin filaments emanate from the surface of vesicles creating an entangled network (green, left). Myosin-V motors generate force on actin (magenta, right) giving rise to forces throughout the network driving random motion of vesicles. (B) We model vesicles embedded in a mechanical continuum with local cage stiffness (κ) represented by the blue harmonic potential, viscoelastic dissipation (ζ_α,α), and thermal fluctuations (ξ) (left). Active processes rearrange the network through bursts of motion (v_A), resulting in displacement of the local cage (blue harmonic), which generates the active force (κζ_αv, red arrow) that drives vesicle motion toward the local minimum (right) (inset indicates active force kinetics). (C) The active force spectrum (S_active) quantifies the forces on vesicles due to only active processes. Combined with our quantitative model we find that the vesicles are subject to 0.4 pN of force, during a power stroke of length Δx ∼ 20 nm and duration 300 μs, resulting in a vesicle velocity of 320 nm/s, which is strikingly similar to the kinetics measured for single molecule myosin-V in vitro and the in vivo vesicle velocity. The solid line is the theoretical fit (Eq. 7), and the dotted line is a −4/3 power law consistent with the cytoplasmic-skeleton mechanics. (D) Simulated vesicle motion (green squares) agrees with experimental data (blue circles) for a wide range of timescales as shown by the probability distribution of displacements. This includes long timescale (t_pdf = 1 s) Gaussian behavior and short timescale (t_pdf = 0.01 s) non-Gaussian tails (indicated by red-shaded regions) that suggest molecular motor behavior, where t_pdf is the time-lag for calculation of the displacement correlations. Gaussian distributions shown in black. These results show that molecular level kinetics of active processes can be extracted from mesoscopic in vivo measurements. To see this figure in color, go online.