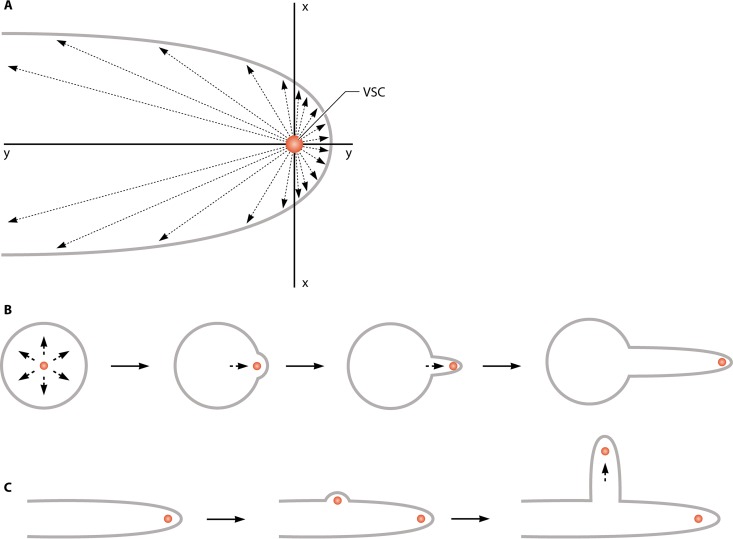
FIG 1.

VSC (vesicle supply center) model for hyphal morphogenesis. (A) The “hyphoid,” a perfect hyphal shape. The hyphoid curve is a geometric function derived from a computer-simulated secretory process, where growth units (vesicles) emanating from a forward-moving source (the VSC) extend the cell surface in a sharply polarized manner. When analyzed mathematically, the process yielded the hyphoid equation y = x cot (x V/N), where N is the amount of cell wall-building vesicles produced per unit of time and V is the rate of advancement of the VSC; when plotted on Cartesian coordinates, the function generates a unique curve that follows closely the actual profile of regular hyphae (Adapted from reference 23.) (B) Displacement and advancement of the VSC from its concentric position in a spore generate a germinating tube. (C) The formation and advancement of a new VSC at a subapical hyphal region generate a lateral branch.