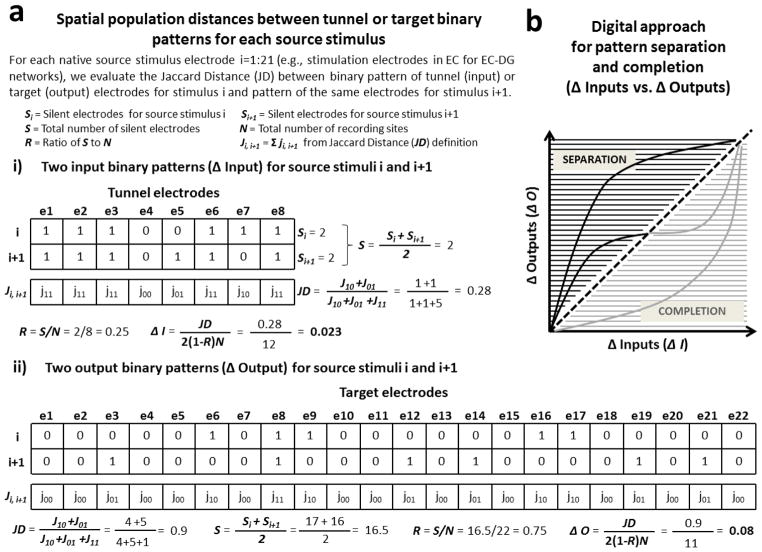
Figure 3.

Digital pattern separation or completion from spatial population distances between binary input and output patterns. (a) Examples of spatial population distance based on Jaccard distance adjusted for sparseness between two binary input patterns (Δ Input, a.i) and between two binary output patterns (Δ Output, a.ii) are shown in the figure. (b) Model adapted from Yassa and Stark (2011) for pattern separation and completion based on spatial population distances of binary input and output patterns (Δ Inputs vs. Δ Outputs). The black portion describes situations in which outputs are more dissimilar than inputs (i.e. separation: Δ Outputs > Δ Inputs). The gray portion describes the reverse situation in which outputs are more similar than inputs (i.e. completion: Δ Outputs < Δ Inputs).