On the origin of molecular oxygen in cometary comae

Introduction

Molecular oxygen was detected¹ in the coma of 67P by the on-board Rosetta Orbiter Spectrometer for Ion and Neutral Analysis (ROSINA)–Double Focusing Mass Spectrometer (DFMS)². It had a high volume mixing of about 1–10%. Primordial O₂ within the nucleus of comet 67P is compatible with the instrumental observations^1,3. Yao and Giapis⁴ proposed an alternative mechanism. The Eley–Rideal (ER) reactions of energetic water-group ions with Si/Fe oxides, or other minerals present on the surface of the nucleus, can generate ${\mathrm{O}}_2^{-}$. Yao and Giapis⁴ suggested that ${\mathrm{O}}_2^{-}$ and energetic O₂ (after photo-detachment) generated through ER reactions can be detected by ROSINA–DFMS and must be an important contributor of the O₂ reported. In this correspondence paper, we do not disregard the fact that ER reactions can happen on the surface of the nucleus. However, we demonstrate that the amounts of ${\mathrm{O}}_2^{-}$ produced through ER reactions cannot explain a significant fraction of the O₂ detected by ROSINA–DFMS. In addition, we find that ${\mathrm{O}}_2^{-}$ and energetic O₂ generated through ER reactions could not be efficiently converted into the ROSINA–DFMS instrument and are therefore not responsible for the reported measurements.

To estimate the maximum amount of oxygen produced through ER reactions, we first consider the amount of ions, produced within the coma, that could impact the comet nucleus. Consistently with the multi-instrument analysis of Rosetta Plasma Consortium (RPC) and ROSINA sensors^5,6, the number density of the expanding gas at a cometocentric distance r is⁷:

$$n\left(r\right)=\frac{Q}{4\pi u{r}^2}$$ 1

where Q is the total outgassing rate and u, the outflow velocity. We assume a maximum global Q to estimate the maximum amount of ions produced at a given time. The global ionization frequency is taken to be ν = 10⁻⁶ s⁻¹, which is an upper-bound based on measurements⁸. The production rate, dP, of ions in each spherical shell of thickness dr is constant:

$$\mathrm{d}P=\nu n\left(r\right)4\pi r^2\mathrm{d}r=\left(\nu Q\mathrm{∕}u\right)\mathrm{d}r$$ 2

Once produced, ions retain the velocity of their parent neutrals for several kilometers⁵. Eventually these ions are picked up by the solar wind and have been observed moving anti-sunward⁹. The impact rate Q^* onto the nucleus of radius r₀ (2 km) for unidirectional motion can thus be estimated as:

$$Q^{*}={\int }_{r_0}^{\infty }\left(\frac{\pi r_0^2}{4\pi r^2}\right)\frac{\nu Q}{u}\mathrm{d}r=\frac{\nu Q{r}_0}{4u}$$ 3

This calculation also holds for random motion of ions (Fig. 1). It is somewhat simplistic but still provides an order of magnitude of the number of ions hitting the nucleus. Furthermore, even coupled with a uniform circular gyration induced by the magnetic field, the amount of ions hitting a spherical target would remain the same. The farther away an ion is, the less-likely it is to return to the target. In addition, the presence of ambipolar electric fields to maintain ion-electron neutrality may repel ions approaching the nucleus¹⁰.

Fig. 1.

Schematic of the ion motion and their probability of returning to the nucleus. Shown for unidirectional motion (left side) and random motion (right side). In both case, the probability for an ion (red dot), created at r, to return to the nucleus is ${\left(\frac{r_0}{2r}\right)}^2$

Let us assume that every collision leads to an ER reaction with 100% yield. The production rate of ${\mathrm{O}}_2^{-}$ through this process is therefore directly approximated by Q^*. We assume a realistic (but slow, to obtain an upper bound) neutral outflow velocity of 500 m s⁻¹. Q^* can be related to $Q_{{\mathrm{O}}_{\mathrm{2}}}$, the total production rate of O₂ observed by ROSINA–DFMS, assuming low O₂ mixing ratio (1%) for our upper-bound estimation¹:

$$Q^{*}=Q_{{\mathrm{O}}_2}^{\mathrm{ER}}\simeq 10^{-6}Q\simeq 10^{-4}{Q}_{{\mathrm{O}}_2}\ll Q_{{\mathrm{O}}_2}$$ 4

The maximum fraction of ${\mathrm{O}}_2^{-}$ anions produced through ER reactions, followed by photo-detachment—process which is associated with small cross sections¹¹—is therefore insignificant with respect to the observed $Q_{{\mathrm{O}}_2}$¹. Furthermore, extrapolating the lab samples⁴ as surrogates of the cometary surface is highly speculative given the nucleus porosity (30–65%¹²) and the presence of C and N bearing refractory species¹³ within the surface composition.

An alternative approach is to compute the energetic ion fluxes that could potentially strike the nucleus. Nilsson et al.⁹ computed 24-h average time series of the cometary energetic ion fluxes measured by RPC-Ion Composition Analyzer (ICA)¹⁴ over the 2-year mission. The maximum cometary energetic ion flux measured was about 10¹³ m⁻² s⁻¹. It leads to a total surface production rate $Q_{{\mathrm{O}}_2}^{ER}$ of ${\mathrm{O}}_2^{-}$ of the order of 10²⁰ s⁻¹. It is still too low to reach volume mixing ratios of 1–10%: even for a low $Q_{{\mathrm{O}}_2}$ (1% mixing ratio of O₂ and low Q of 10²⁶ s⁻¹)^1,7, $Q_{{\mathrm{O}}_2}^{ER}$ is about $10^{-4}{Q}_{{\mathrm{O}}_2}$.

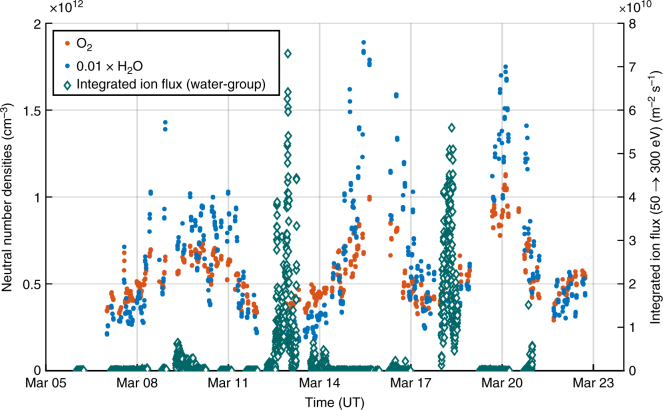
Figure 2 shows a time series of the energetic cometary water-group ion fluxes measured by RPC–ICA close to the nucleus from 5 to 23 March 2016. After correction for the negative spacecraft potential measured by the Rosetta dual Langmuir Probe (RPC–LAP)¹⁵, the flux is integrated over the 50–300 eV energies that are efficient to trigger the ER reactions⁴. The maximum value is 10¹¹ m⁻² s⁻¹. The number densities of O₂ and H₂O derived from ROSINA–DFMS and ROSINA–COPS are over-plotted in Fig. 2. The fluctuations are essentially seasonal¹⁶ with high outgassing over the summer hemisphere and low outgassing over the winter hemisphere. There is a clear anti-correlation between O₂ densities and the energetic ion fluxes, drivers of ER reactions. With a high outgassing, the flux is weakened by the time it reaches the nucleus due to ion-neutral collisions. The highest neutral densities of O₂ are therefore not driven by energetic ions through ER reactions on the surface. In addition, O₂ and H₂O are strongly correlated¹⁶ (Fig. 2) and follow a r⁻² Haser law⁷. This is incompatible with an extended source of O₂ through ER reactions that could happen on dust grains or the spacecraft itself.

Fig. 2.

Time series of oxygen and water neutral number densities. Time series of the O₂ (orange) and H₂O (blue) neutral number densities (left y-axis) from March 6 to 23, 2016. The spacecraft was located at about 10 km from the nucleus. Note that the H₂O neutral densities have been multiplied by 10⁻² to fit on this scale. Time series of the total RPC–ICA energetic water-group ions (H₂O⁺, H₃O⁺, OH⁺) of cometary origin (green), corrected for the RPC–LAP spacecraft potential¹⁵ and integrated over the effective energy range of the Eley–Rideal reactions (50–300 eV)⁴ (right y-axis)

As for the instrumental detections, Yao and Giapis⁴ claim that ${\mathrm{O}}_2^{-}$ and energetic O₂ may hit the gold-coated surfaces of the ROSINA-DFMS and be efficiently converted into ${\mathrm{O}}_2^{+}$. ROSINA–DFMS² has two operation modes (neutral and ion) which differ by only the electrostatic bias and filament emission. In neutral mode, the ionization box is biased relative to spacecraft potential by +200 V to keep primarily cometary ions out and the filament emits electrons to ionize incoming neutrals. In ion mode, ROSINA–DFMS primarily measures thermal ions with no bias voltage on the ionization box and the filament is heated below the electron emission threshold (sub-emission). The angular acceptance of the electrostatic analyser is 5° and the energy acceptance is 1% of the nominal ion energy which depends on the mass measured. For mass 32 Da, this voltage is −1900 V: only species with energies below 19 eV pass the electrostatic analyser.

In neutral mode, ${\mathrm{O}}_2^{-}$ anions with energies higher than the spacecraft potential (varying between 0 and −30 V¹⁵) would enter the instrument and be accelerated to about 200 eV. The impact of the gold surface to convert into ${\mathrm{O}}_2^{+}$ would have to be accompanied by the loss of the 200 eV with minimal scattering to fit into the narrow angular acceptance angle. It is rather unlikely.

In the case of energetic O₂, following the suggested conversion to ${\mathrm{O}}_2^{+}$ via collisions with the gold surface, these ions, with energy below 19 eV and minimal angular scattering (<5°), could enter the sensor equally well in neutral and ion modes. As such, the peak height for mass 32 Da would be the same in both modes. In neutral high resolution mode, the peak was continuously strong, normally exceeding 10⁴ ions. However, in the ion mode, the signal was below detection limit for mass 32 Da in high resolution. This precludes the possibility that ${\mathrm{O}}_2^{-}$ and energetic O₂ are efficiently ionized in the DFMS sensor by hitting the surfaces of the instrument. These observations are much more consistent with a thermal neutral O₂ population, partially ionized⁸. If the ER mechanism is in action, its contribution to the O₂ peak in the neutral mode is very minor.

Conclusions

We have used generous assumptions in terms of production rates and ion fluxes to assess the production of ${\mathrm{O}}_2^{-}$ through ER reactions as a mechanism to explain Rosetta observations. Even with these assumptions, the amount of O₂ produced is insignificant (by several orders of magnitude) with respect to what was detected by ROSINA–DFMS. There are not enough ions in the coma and the series of events required to trigger these processes are individually too rare. Furthermore, cometary ion fluxes are anti-correlated to the O₂ densities observed by ROSINA. Finally, in terms of the instrument itself, there is little evidence of the production and detection of products ${\mathrm{O}}_2^{-}$ and energetic O₂ by ROSINA–DFMS. While ER reactions may occur, they cannot explain the amounts of O₂ detected. Primordial O₂^1,3 remains compatible with the quantities and trends of molecular oxygen measured by ROSINA–DFMS, while other theories¹⁷ discuss other plausible sources.

Author contributions

H.N., A.I.E., P.H., and C.M.C., as Principal Investigators of RPC; K.A., as Principal Investigator of ROSINA; and M.G.G.T., as Rosetta Project Scientist, initiated the project to write a correspondence paper on this topic, based on Rosetta in-situ measurements. K.L.H. coordinated the team effort and carried out the manuscript preparation. K.A., J.-J.B., C.M.C., J.D.K., A.I.E., S.A.F., M.G., T.I.G., P.H., F.L.J., H.N., M.R., C.S.W., M.G.G.T., and E.V. contributed to the manuscript preparation. K.L.H., M.G., and A.B. computed an upper-bound for the ion-nucleus collisions. C.S.W. and H.N. analyzed the RPC–ICA dataset and derived the energetic ion fluxes used in Fig. 2. F.L.J. and A.I.E. analyzed the RPC–LAP dataset used to correct the ion fluxes for the spacecraft potential. K.A. and M.R. analyzed the ROSINA–DFMS dataset shown in Fig. 2. C.M.C., A.I.E., P.H. and H.N. contributed to the development and operation of the RPC sensors. K.L.H., A.B., A.I.E., M.G., P.H., F.L.J., H.N., C.S.W. and E.V. contributed to the interpretation of the RPC dataset. K.A., M.R., J.-J.B., J.D.K., S.A.F. and T.I.G. contributed to the development and operation of the ROSINA–DFMS instrument and to the interpretation of its dataset.

Competing interests

The authors declare no competing interests.