Figure 2.

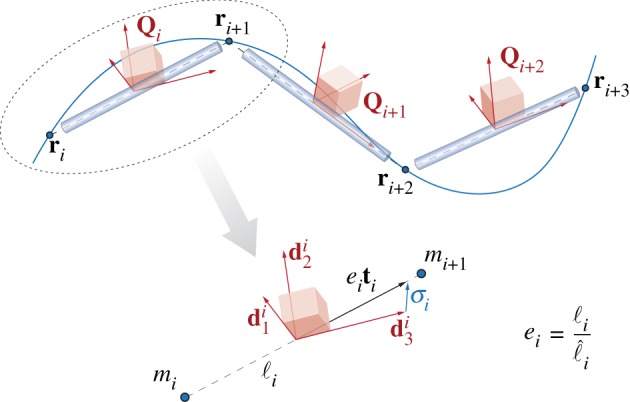
Discretization model. A discrete filament is represented through a set of vertices r(t)_i=1,…,N+1 and a set of material frames ${\mathbf{\text{Q}}}_i(t)={\{{\mathbf{\text{d}}}_1^i,{\mathbf{\text{d}}}_2^i,{\mathbf{\text{d}}}_3^i,\}}_{i=1,\dots ,N}$. Two consecutive vertices define an edge of length ℓ_i along the tangent unit vector t_i. The dilatation is defined as $e_i={\ell }_i/{\hat{\ell }}_i$, where ${\hat{\ell }}_i$ is the edge rest length. The vector ${\mathit{\sigma }}_i=e_i{\mathbf{\text{t}}}_i-{\mathbf{\text{d}}}_3^i$ represents the discrete shear and axial strains. The mass m_r of the filament is discretized in pointwise concentrated masses $m_{i=1,\dots ,n+1}$ at the locations r_i for the purpose of advecting the vertices in time. For the evolution of Q_i in time, we consider instead the mass second moment of inertia ${\widehat{\mathbf{\text{J}}}}_{i=1,\dots ,n}$ associated with the cylindrical elements depicted in blue.