Figure 1.

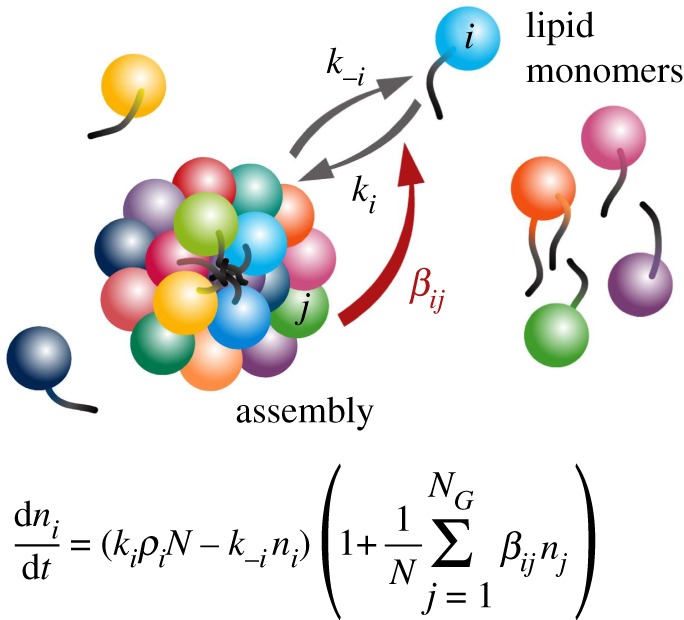
The graded autocatalysis replication domain (GARD) model is based on computer simulations of rigorous chemical behaviour. The model involves a stochastic chemistry simulation based on a set of differential equations as shown. The main reaction step is the entry and exit of an amphiphilic molecule A_i, belonging to a repertoire of N_G amphiphile types (represented by different colours), between the environment and an assembly (in this figure exemplified by a small micelle). The variable n_i is the count of A_i molecules within the assembly, N = Σn_i, the total count of all N_G species in the assembly, k_i and k_−i are, respectively, the basal (spontaneous) forward and backward rate constants for A_i, (black arrows), and ρ_i is the external concentration of A_i. A key aspect, crucial for reaching a kinetically controlled homeostatic growth of the assembly, is the dependence of the reaction rates on the current composition of the assembly. This dependence is controlled by a matrix β, whose elements β_ij are the rate-enhancement values for internal compounds on the rate of the exchange reaction. The matrix element β_ij signifies the rate-enhancement parameters for the catalysis exerted by the in-assembly species A_j on the joining and leaving reactions of A_i (red arrow). The matrix elements thus control the dynamics of the mutually catalytic network embodied in the GARD assembly, and its elements are drawn from a probability distribution generated through the RAD model (§4) [65].