Figure 3:

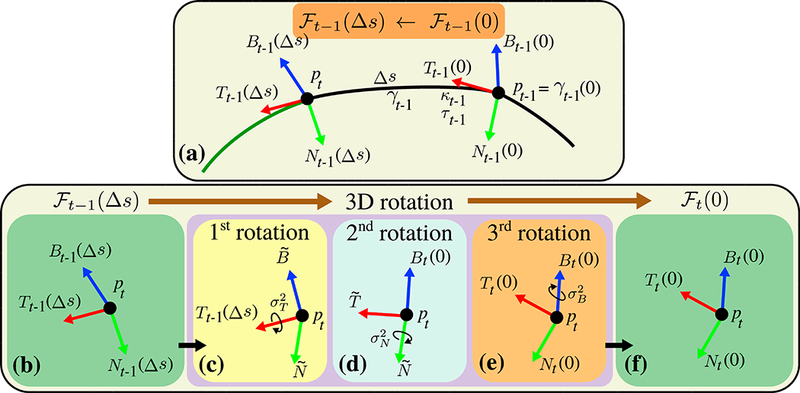
(a) Frenet-Serret frame rotates as a function of arc length, s. The frame at p_t-1, ℱ_t-1(0), gradually changes into the frame at p_t-1, ℱt_-1(Δs), during propagation. At p_t, based on ℱ_t-1(Δs), the Frenet-Serret frame of a candidate curve, ℱ_t (0), is randomly generated using 3 consecutive rotations. (b-c) ℱ_t-1(Δs) is first rotated around T_t-1(Δs) with a random angle picked from a zero mean Gaussian distribution with user defined variance ${\sigma }_T^2$, creating $\tilde{N}$ and $\tilde{B}$ vectors. (d-e) Second and third rotations are done around $\tilde{N}$ and B_t(0) with random angles picked from zero mean Gaussian distributions with user defined variances ${\sigma }_N^2$ and ${\sigma }_B^2$ respectively. Notice that the Frenet-Serret frame is always orthonormal during the propagation and after each rotation.