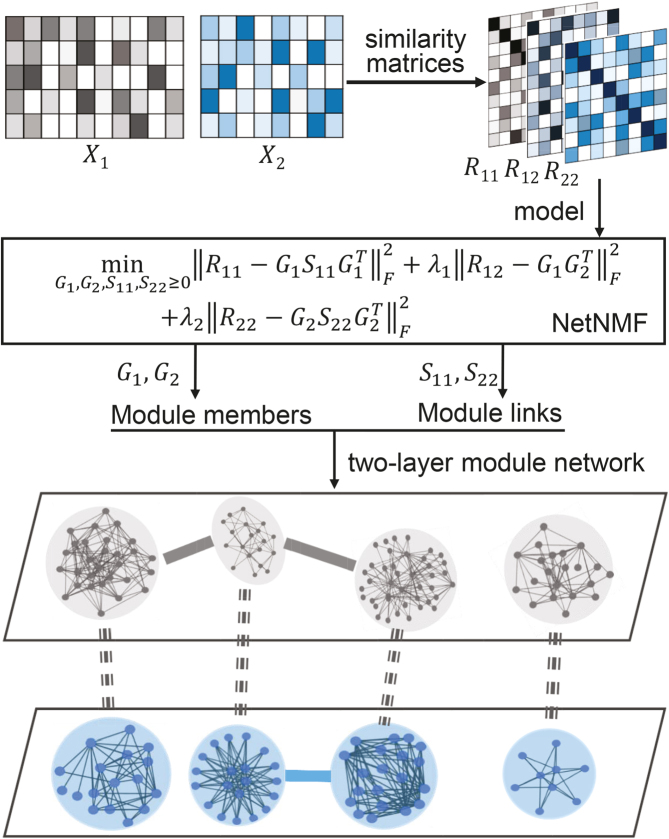
Figure 1.

Overview of the NetNMF for discovering a two-level module network by integrating pairwise genomic data. Three matrices R₁₁, R₁₂, R₂₂ are computed via Pearson correlation, representing the similarities within and between two types of features in the pairwise input data matrices X₁ and X₂. NetNMF simultaneously decomposes R₁₁, R₁₂, R₂₂ to get the underlying co-modules and their associations. The ith co-module is identified based on the ith column vector in factored matrices G₁ and G₂; the association degree between the ith and jth modules is determined by S₁₁(i, j) (or S₂₂(i, j)), where S(i, j) represents the element of the ith row and jth column in this matrix. Thus, a two-layer module network could be constructed in which a node represents a module.