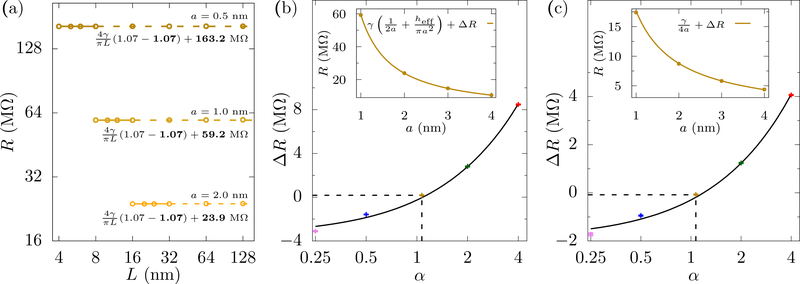
FIG. 4.
Universality of the golden aspect ratio with pore size. (a) The resistance versus L for cylindrical cells with α = 1.07, hp = 1 nm, γ = 71 MΩ·nm, and various pore radii. The golden aspect ratio is fairly constant over this range of a. The solid lines show the fits and the dashed lines show the extrapolations – which make good predictions of R at large L. The fit parameters are shown in bold. The errors of the fits for f’s and R∞’s are less than 0.5 % and 0.1 % respectively. As the pore radius increases, we examine fits for larger L, to roughly keep proportionality between L and a as there are (non-scaling) finite-size effects that will affect the simulations when the radius starts to become comparable to the cell cross-sectional length. The range of the fitting can influence the extracted f (we expect that ultra-precise calculations at really large L will reveal the exact golden aspect ratio, which will be close to 1.07). (b) ΔR from fits to for cylindrical cells of various α, with heff and ΔR as fitting parameters. We get ΔR ≈ 0 for the “golden aspect ratio” α★= 1.07 (dashed lines), the fit of which is shown in the inset. The effective height, heff, is (5±1) % larger than the 1 nm membrane thickness, see Fig. 5. (c) ΔR in the fits R = γ/4a +ΔR for half cylinders of different aspect ratios with the pore mouth set at potential V = 0. We again get ΔR ≈ 0 for α★ = 1.07 (dashed lines), whose fit is shown in the inset. The interpolated lines in the insets are for visual clarity only.