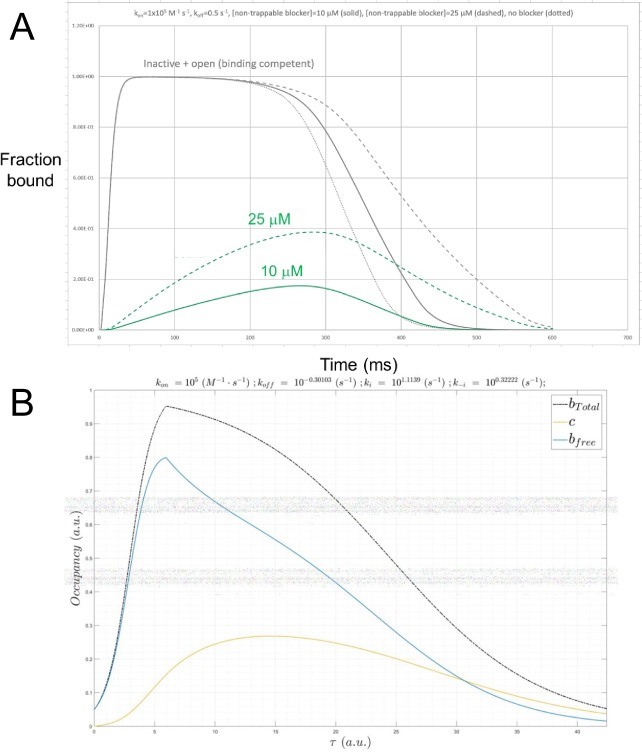
Fig 14.

(A) Plot of dynamic fractional hERG occupancy in mid-myocardial (M) cells by a hypothetical non-trappable blocker exhibiting k_on = 1x10⁵ M^-1 s^-1 and k_off = 5x10^-1 s^-1 (K_d = 5 μM and $K_d^{\prime }$ = 20 μM) sampled at 10 and 25 μM (green solid and dashed curves, respectively). The total dynamic binding site level consists of the sum of the open/conducting and inactivated channel populations (gray dotted, solid, and dashed curves corresponding to 0, 10, and 25 μM blocker concentrations, respectively). The overall shape of the channel state population curves qualitatively resembles our logistic model (in which k_i is very fast), noting that recovery from inactivation (the decay region of the curves) is slowed by blockade due to the smaller contribution of the hERG current to the membrane potential, which in turn, alters the response of the channel population (consistent with the recursive effect depicted in Fig 2). The blocker occupancy curves reflect nSSO occupancy at k_on = 1x10⁵ M^-1 s^-1 (where $k_{on}<<k_{o{n}_{SS}}$, as suggested from Table 1), and the peak occupancy is far below 50% at 5 μM. Nevertheless, even transient fractional occupancy approaching the ~45% level can be highly pro-arrhythmic [4]. (B) Plot of B_total(τ) (gray), B_free(τ) (blue) and c(τ) (gold) simulated using our logistic model (scenario 2) for a hERG blocker exhibiting K_d = 5 μM (k_on = 1x10⁵ M^-1 s^-1 and k_off = 0.5 s^-1) and [free blocker] = 10 μM. k_i and k_−i were set to 13 s^-1 and 2.1 s^-1, respectively, so as to reproduce B_max at τ ≈ 50 ms and Λ ≈ 350 ms. B_total(τ) and c(τ) are qualitatively similar to the curves in A.