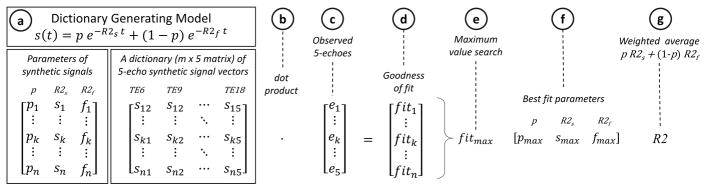
Figure 2.

Overview of the dictionary search method to estimate the slow R2 component (R2_s), fast R2 component (R2_f), and slow-component fraction (p) for the time-dependent signal intensities S(t) (equation, a). For a given 5-TE spin-echo imaging protocol, a dictionary of a large number (n) of unit-length synthetic signal vectors (an n × 5 matrix) is calculated a priori, using a dictionary-generating model on a 3-dimensional grid: p = [0.1:0.01:0.3]; R2_s = [1:1:100] s⁻¹; and R2_f = R2_s + Δ, where Δ = [1:1:250] s⁻¹ (a). Each row of this dictionary is a signal vector with a different signal model parameter combination (p, R2_s, R2_f). (b & c) An observed 5-TE signal vector at each pixel location is compared to all synthetic signal vectors in the dictionary by matrix-vector dot product operation. (d) The resulting dot product values serve as goodness-of-fit metric. (e) A search for the maximum goodness-of-fit value identifies the synthetic signal “closest” to the observed signal, i.e. “the best fit.” (f) The best-fit model parameters are extracted, and (g) the final R2 value is calculated as a weighted average of R2_s and R2_f.