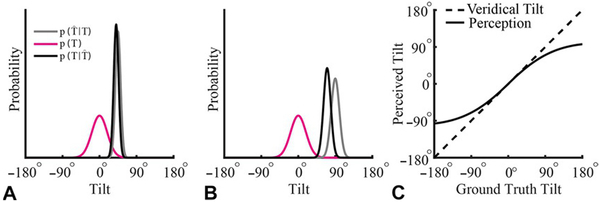
Fig. 3.4.

Bayesian account of the Aubert effect. (A, B) Tilt estimation for ground truth tilts of 45° and 90° relative to the world, respectively. A prior for being upright relative to the world (tilt = 0°), p(T), is illustrated in magenta. The precision of sensory representations of tilt decrease the further an individual is from upright. Reflecting this change in precision, the sensory likelihood function $p(\widehat{T}|T)$ representing a tilt of 45° is taller and narrower (i.e., more precise) than for a tilt of 90° (gray curves). With Bayesian cue integration, the prior has a larger effect on the posterior, $p(T|\widehat{T})$ (black curves), at larger tilts because of the decreased precision of the likelihood function. Correspondingly, the posterior is “pulled” more towards the prior in (B) compared to (A). (C) Taking the tilt with the highest probability as the estimate of the ground truth tilt, this “pull” on the posterior introduces a bias in the perceived tilt that increases the further the individual is from upright. Specifically, the perceived tilt (solid curve) is underestimated at large tilts (dashed curve), resulting in the Aubert effect.(Adapted from De Vrijer M, Medendorp WP, Van Gisbergen JAM (2008) Shared computational mechanism for tilt compensation accounts for biased verticality percepts in motion and pattern vision. J Neurophysiol 99: 915–930.)