Figure - PMC

Skip to main content

An official website of the United States government

Here's how you know

Here's how you know

Official websites use .gov
A .gov website belongs to an official government organization in the United States.

Secure .gov websites use HTTPS
A lock ( ) or https:// means you've safely connected to the .gov website. Share sensitive information only on official, secure websites.

View full-text article in PMC

. 2018 Nov 22;29(2):875–891. doi: 10.1093/cercor/bhy291

Search in PMC
Search in PubMed
View in NLM Catalog
Add to search

© The Author(s) 2018. Published by Oxford University Press.

This is an Open Access article distributed under the terms of the Creative Commons Attribution Non-Commercial License (http://creativecommons.org/licenses/by-nc/4.0/), which permits non-commercial re-use, distribution, and reproduction in any medium, provided the original work is properly cited. For commercial re-use, please contact journals.permissions@oup.com

PMC Copyright notice

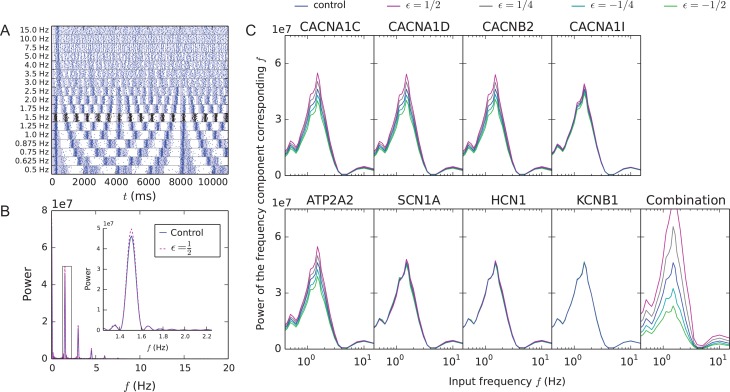
Figure 2. — Model variants affect the magnitude of the response of the network to oscillatory inputs. (A) Population spike trains for control networks where the event times of the background synaptic inputs were non-stationary (oscillatory) Poisson processes. The shaded areas show the times at which the λ of the oscillatory Poisson process was larger than average. The black spike train represents the L5PC network response to the input oscillation frequency 1.5 Hz, which caused the largest response in the network. (B) Fourier power spectra of the CACNA1C model variant (purple) and control (blue) network spike train with the input oscillation frequency 1.5 Hz. Only spikes from 2000 ms onward were considered when calculating these spectra. Inset: zoomed-in view on the peak at the frequency corresponding to the input oscillation. (C) The power of the frequency component corresponding to the input oscillation frequency (e.g., the amplitude of the peak in the inset of panel B) plotted against the input oscillation frequency. Averaged over 6–13 (mean 11.9) samples. Background oscillation frequencies f = 0.0625, 0.125, 0.1875, 0.25, 0.3125, 0.375, 0.4375, 0.5, 0.5625, 0.625, 0.6875, 0.75, 0.875, 1.0, 1.125, 1.25, 1.375, 1.5, 1.625, 1.75, 2.0, 2.5, 3.0, 3.5, 4.0, 5.0, 7.5, 10.0 and 15.0 Hz were considered. Blue: control network (no variants), other colors: downscaled model variants with different downscaling parameters ε.