Skip to main content
. 2019 Jan 8;116(3):454–468. doi: 10.1016/j.bpj.2019.01.003

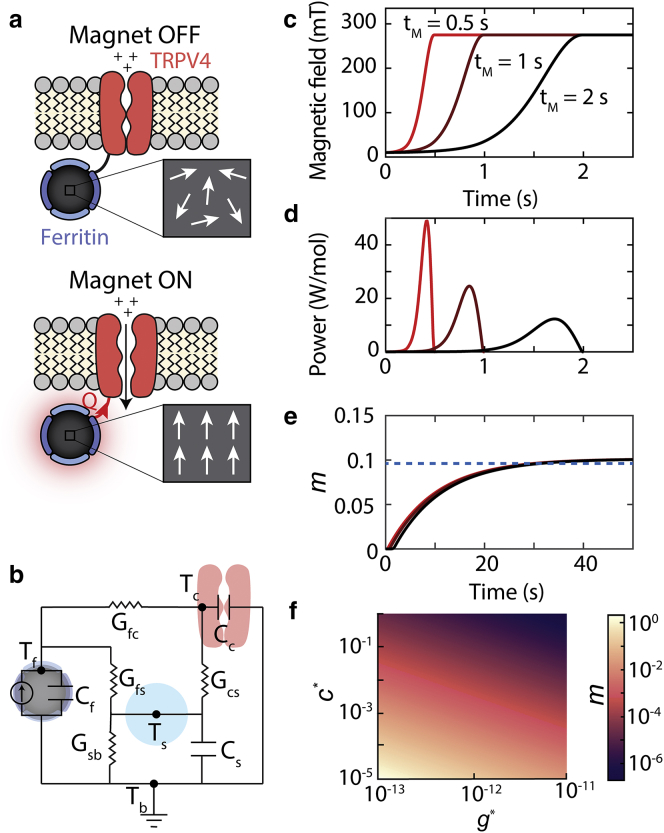
Figure 3.

Figure 3

The magnetocaloric gating mechanism: (a) schematic shows how the magnetocaloric effect in ferritin can activate nearby temperature-sensitive ion channels (e.g., TRPV4). An applied magnetic field will align the magnetic moments within paramagnetic ferritin nanoparticles, which will reduce the magnetic entropy. The reduced magnetic entropy generates heat (Q) via the magnetocaloric effect that can activate a nearby temperature-sensitive ion channel. Here, we have depicted ferritin as a paramagnet, but the calculations are equivalent for superparamagnetic particles. (b) The equivalent circuit model used to estimate heat transfer between the ferritin particle and ion channel is shown. Tf, Tc, Ts, and Tb represent the temperature of the ferritin, channel, water shell around ferritin, and bath, respectively. Cf, Cc, and Cs represent the heat capacities of the ferritin, channel, and near-water shell, respectively. Gfc, Gfs, Gcs, and Gsb represent the thermal conductances between the ferritin and channel, ferritin and water shell, channel and water shell, and water shell and bath, respectively. (c) The applied magnetic field as a function of time for three different magnetization times (tm = 0.5, 1, and 1.5 s) is shown. (d) The power generated in a mole of ferritin particles because of magnetocaloric effect for the magnetic field profiles in (c) is shown. (e) The number of additional openings per channel (m) caused by the magnetocaloric effect based on (d) and Eq. 5 is shown. The dashed blue line indicates the maximal percentage of channels that open as derived by the analytical expression for m in Eq. 8. Note that the total number of channels that open depends on the maximal value of the magnetic field and not the rate of magnetization. Calculations assume Tb = 25°C, c∗ = 10−5, and g∗ = 10−12. (f) The fraction of channels that respond depends on the value of c∗ (heat capacity scaling factor) and g∗ (thermal conductance scaling factor), which can vary by orders of magnitude depending on the biophysical mechanism that triggers temperature-dependent channel gating. We expect that the m values near 10−5 and greater would yield a physiological response.