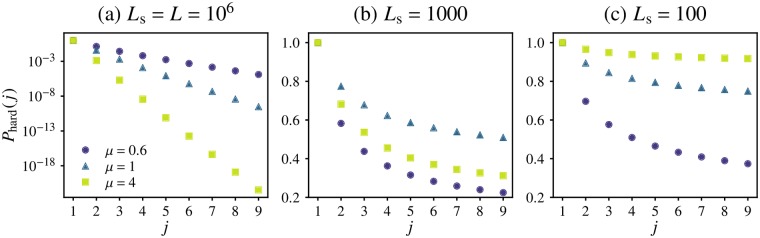
Fig 8. Dispersal promotes soft sweep detection in small subranges.

(a)–(c) Probability of observing a hard sweep in j samples randomly chosen from contiguous subranges of different sizes L_s in simulated 1D ranges of size L = 10⁶, with rescaled mutation rate $\tilde{u}={10}^{-6}$. At the same mutation rate, broader dispersal kernels lead to a larger average clone size (〈X〉 ≈ 980, 1.6 × 10⁴, 4 × 10⁴ for μ = {4, 1, 0.6} respectively), which reduces the total number of alleles and favours hard sweep signatures when the sampling is done over the entire range [L = L_s, (a)]. However, when L_s is reduced [(b)–(c)], the detection of soft sweeps become increasingly likely for the broader dispersal kernels; the broken-up structure of clones compensates for their smaller overall number. For small enough subranges, the order of values of P_hard(j) with increasing μ is inverted compared to the values at L_s = L.