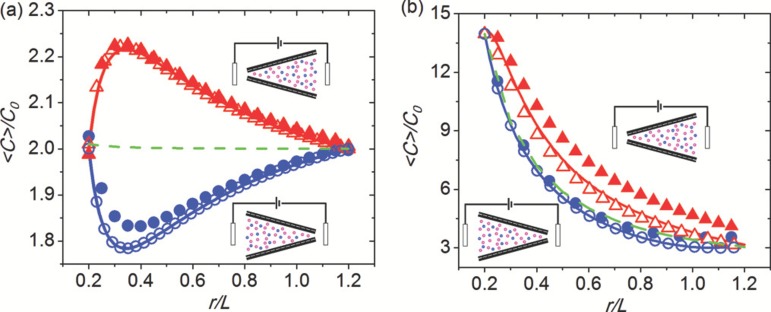
FIG. 4.

Comparison of the theoretical result from an asymptotic theory for the zero cone angle limit to computed results from the full Poisson-Boltzmann equation. The theoretical and computed cross-section averaged total ion concentration distribution $⟨c⟩$, normalized by the bulk concentration $c_0$, is plotted against the radial coordinate from the (imaginary) cone tip normalized by the pore length $L$. The concentration distribution is observed to be very different for opposite polarization at $FV/R_{g}T=10$ and for weakly and strongly selective nanopores, as defined by a dimensionless parameter $X$ (see Ref. 126): the ratio of the surface charge concentration divided by the pore radius, to $c_0$. The total concentration for Donnan equilibrium (green dashes), namely, when V = 0, is also given for comparison. The computation is done by solving both 1D (open symbol) and 2D (closed symbol) Poisson-Nernst-Planck equations. Enrichment occurs when applying a voltage from tip to base (positive V${}_0$, red, lines, and up-triangles) and depletion with reverse bias (blue, lines, and circles). The tip radius, base radius, length, and surface charge density of nanopore are fixed at 5 nm, 30 nm, 1 $\mu$m, and 0.05 C/m${}^2$, respectively. The size of the reservoirs for 2D is 500 nm $\times$ 500 nm. A symmetric electrolyte KCl with the diffusion coefficient of 2 $\times {10}^{-9}$ m${}^2$/s is used. (a) Weakly selective, the concentration is 1000 mM (X${}_{\mathrm{tip}}$ = 0.21). (b) Strongly selective, the concentration is 15 mM (X${}_{\mathrm{tip}}$ = 13.8). Adapted with permission from Yan et al., J. Chem. Phys. 138, 044706 (2013). Copyright 2013 AIP Publishing LLC.