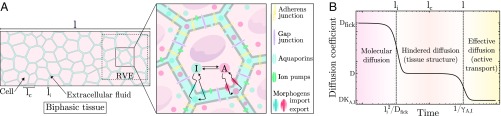
Fig. 1.

Model for pattern formation in active biphasic tissues. (A) Schematic of the model. (Left) Cells form a poroelastic network, permeated by extracellular fluid, where three natural length scales can be defined: the interstitial space size ($l_i$), the characteristic cell size ($l_c$), and the tissue size ($l$). (Right) Biochemical interactions between morphogens, $A$ and $I$, take place inside the cell and are described by their respective turnover rate functions $f\left(A,I\right)$ and $g\left(A,I\right)$. $A$ and $I$ are exported across the cell membrane at rates ${\lambda }_{A,I}$ and imported at rates ${\gamma }_{A,I}$, respectively. In the extracellular space, both $A$ and $I$ spread freely by diffusion at the same rate $D$ or can be advected by the fluid at velocity $v_e$. (B) Evolution of the effective diffusion coefficient as a function of time and space scales. At shorter distances and times, diffusive behavior of morphogens is described by a molecular diffusion coefficient, $D_{\text{Fick}}$. At intermediate scales, the diffusive motion of morphogens starts to be hindered by cells and the global diffusion coefficient, $D$, depends of the tissue spatial organization through ${\varphi }^{*}$. At larger scales, morphogen diffusion is controlled by dynamic interactions with cells (import/export, adsorption/desorption,) and an effective coefficient $D{K}_{A,I}$ (9).