Figure - PMC

Skip to main content

An official website of the United States government

Here's how you know

Here's how you know

Official websites use .gov
A .gov website belongs to an official government organization in the United States.

Secure .gov websites use HTTPS
A lock ( ) or https:// means you've safely connected to the .gov website. Share sensitive information only on official, secure websites.

View full-text article in PMC

. 2019 Mar 27;9:5282. doi: 10.1038/s41598-019-41503-8

Search in PMC
Search in PubMed
View in NLM Catalog
Add to search

© The Author(s) 2019

Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.

PMC Copyright notice

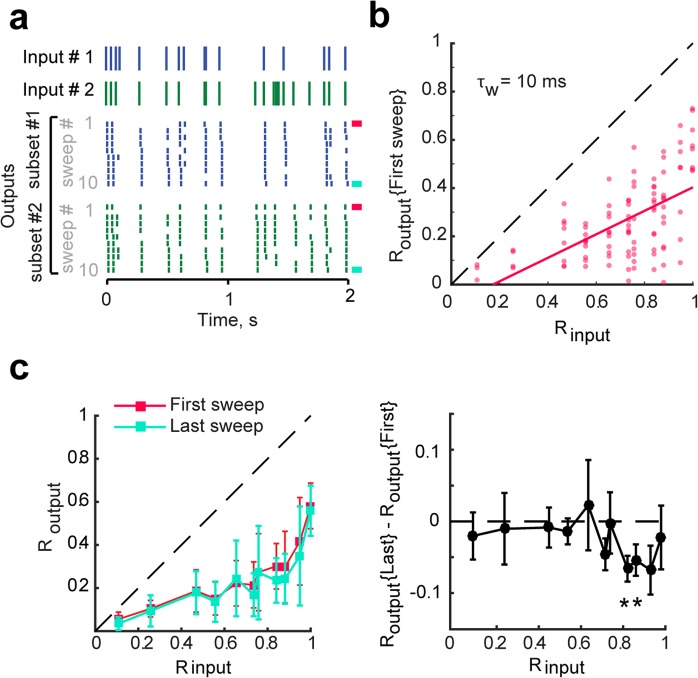
Input spiketrains are efficiently separated upon their first presentations. (a) Two of five inputs are shown with corresponding output spiketrains. The first output sweep is marked with a pink bar (right) and last sweep is marked with a blue bar. (b) R_output, computed from the first sweep of five output trains only (pink), as a function of R_input, with linear fit. All data points are below the identity line indicating that outputs are effectively decorrelated compared to their inputs even when input patterns have only been presented once each. The average decorrelation (R_input − R_output) is significant for all input sets (one-sample T-tests, p < 0.01) except for R_input = 0.11 (p = 0.1). (c) Left: Output correlations (Mean ± SEM) between spiketrains of the first sweep (pink) and the last sweep (blue). There is no significant difference (ANCOVA, p = 0.33). Right: When taking into account that the two distributions are paired, we detect that a few output correlations are significantly lower for the last sweep than for the first one (one-sample T-test on the difference between R_output of the first and last sweep of each recording set, asterisks signify p < 0.05). This is evidence, though weak, that repetition of input spiketrains might improve pattern separation for highly similar inputs.