Figure 1.

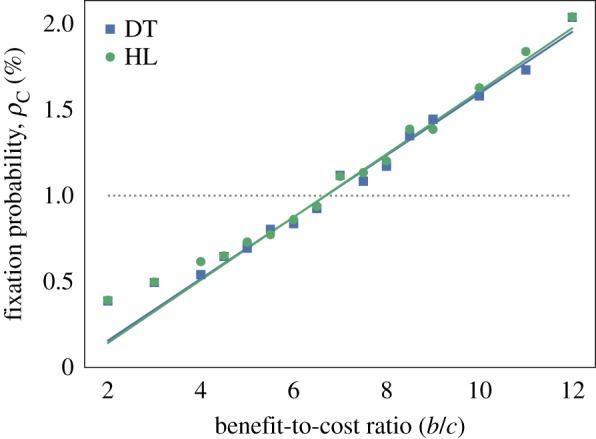
Fixation probabilities for a Prisoner’s Dilemma game in the EGT model with c = 1 and δ = 0.025. Solid lines plot theoretical fixation probabilities for a single cooperator on a hexagonal lattice (HL, green) and a Delaunay triangulation (DT, blue), obtained from equation (2.5). The critical benefit-to-cost ratio, which occurs where fixation probability is equal to ρ₀ = 1/N (grey, dotted line.), is (b/c)* ≈ 6.7 for the HL and DT. Simulation results are also shown for both cases and fit well with the theoretical fixation probabilities when (b/c) > 4. However, as equation (2.5) was derived in the weak selection limit we only expect it to be accurate near the critical ratio. (Online version in colour.)