Figure 3.
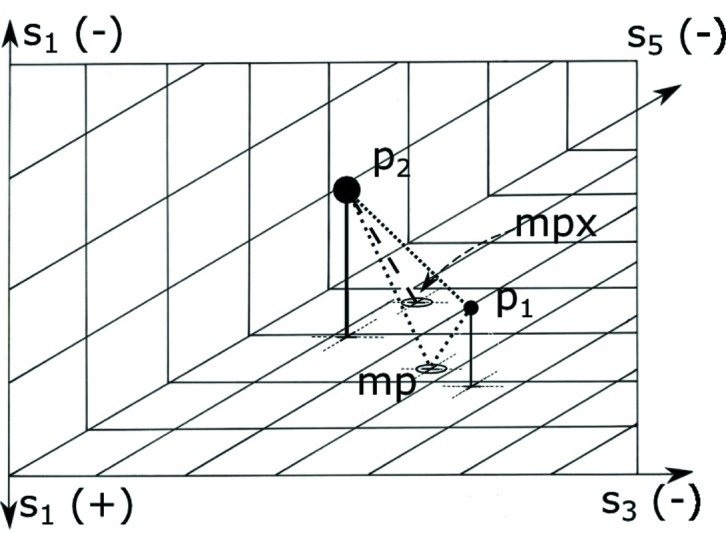
This an example of a one-boundary tunneled mirrored boundary distance calculation. As with Fig. 1 ▸ the 24 reflections are not shown. Both points 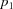 and
and 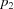 are Selling reduced. The image is the three-dimensional, all-negative octant of the three
are Selling reduced. The image is the three-dimensional, all-negative octant of the three 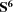 axes,
axes, 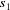 ,
, 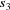 , and
, and 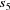 ; the reduction is done along the
; the reduction is done along the 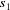 axis, and
axis, and 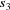 and
and 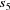 are the two scalars that will be interchanged. The points are shown above the
are the two scalars that will be interchanged. The points are shown above the 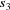 /
/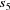 plane, with their projections onto that plane marked with a circled ‘X’. The Euclidean distance from
plane, with their projections onto that plane marked with a circled ‘X’. The Euclidean distance from 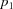 to
to 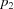 is shown as a dotted line. Let mp be the mirror point on the boundary going from
is shown as a dotted line. Let mp be the mirror point on the boundary going from 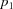 to
to 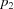 via the boundary. Then the shortest distance from
via the boundary. Then the shortest distance from 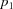 to mp to
to mp to 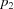 is also shown as a dotted line. The transformed image of mp is mpx. The distance between
is also shown as a dotted line. The transformed image of mp is mpx. The distance between 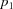 and mp is the same as the distance between a transformed
and mp is the same as the distance between a transformed 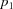 and mpx. There is a no-cost tunnel from mp to mpx. So the total alternative distance for this case is the distance between
and mpx. There is a no-cost tunnel from mp to mpx. So the total alternative distance for this case is the distance between 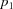 and mp plus the distance from mpx to
and mp plus the distance from mpx to 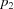 (shown as a dashed line).
(shown as a dashed line).
