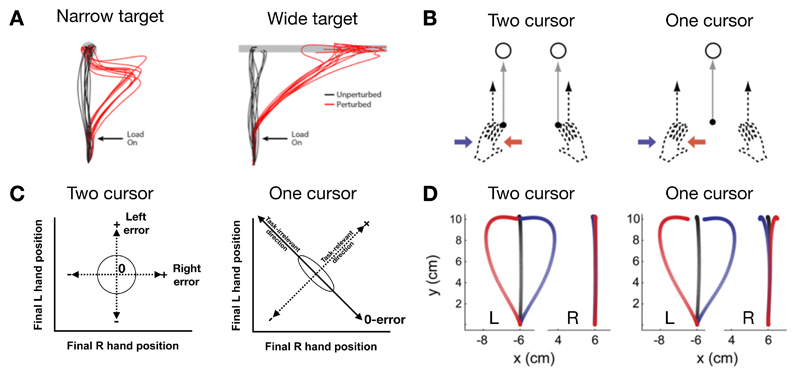
Figure 2. Minimum intervention principle and exploitation of redundancy.

A. Unperturbed movements (black traces show individual hand movement paths) to narrow or wide targets tend to be straight and to move to the closest point on the target. Hand paths during the application of mechanical loads (red traces in response to a force pulse that pushes the hand to the right) delivered immediately after movement onset, which disrupt the execution of the planned movement, obey the principle of minimum intervention. That is, for a narrow target (left), the hand paths correct to reach the target whereas, for a wide target (right), there is no correction and the hand just reaches to another point on the target. B. Participants make reaching movements to targets. In a two-cursor condition, each hand moves its own cursor (black dots) to a separate target. In a one-cursor condition, the cursor is displayed at the average location of the two hands and participants reach with both hands to move this common cursor to a single target. During the movement, the left hand could be perturbed with a leftward (red) or rightward (blue) force field or remain unperturbed (black). C. When each hand controls its own cursor there is only one combination of final hand positions for which there is no error (center of circle). Optimal feedback control predicts that there will be no correlation between the endpoint positions (black circle shows a schematic distribution of errors). When the two hands control the position of a single cursor, there are many combinations of final hand positions which give zero error (black diagonal line; task-irrelevant dimension). Optimal control predicts correction in one hand to deviations in the other leading to negative correlations between the final locations of the two hands, so that if one hand is too far to the left the other compensates by moving to the right (black ellipse). D. Movement trajectories shown for the left and right hand for perturbations shown in B (one-cursor condition). The response of the right hand to perturbations of the left hand shows compensation only for the one-cursor condition in accord with the predictions of optimal feedback control. In addition, negative correlations in final hand positions can be seen in unperturbed movements for the one-cursor but not two-cursor condition (not shown). Modified with permission from [52] (A) and [53] (B-D).