Fig. 4.

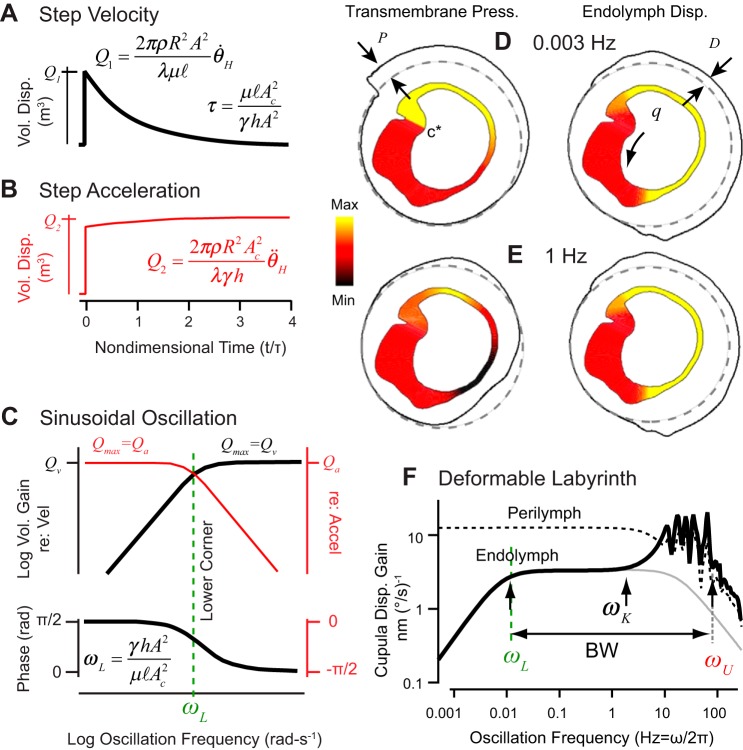
Semicircular canal macromechanics. A and B: rigid labyrinth model prediction for transient cupula volume displacement in response to a step excitatory increase in angular velocity (c.f. Fig. 3C) and for sustained cupula volume displacement in response to a step angular acceleration. C: bode plots showing cupula volume displacement gain and phase as a function of frequency for sinusoidal oscillation of the head. Left axes show magnitude and phase relative to angular head velocity (flat gain above ω_L), while right axes are relative to angular head acceleration (flat gain below ω_L). D and E: pressure and endolymph displacement as a function of position in the horizontal canal membranous duct for sinusoidal rotation at 0.003 Hz (D) and at 1 Hz (E) (yellow: max; red: zero; and black: min). F: membranous duct deformability alters the high-frequency cupula displacement (thick black) relative to a perfectly rigid canal (gray). Deformability increases the bandwidth (BW) by shifting ω_U up and increases high-frequency sensitivity through the role of perilymph deforming the canal. [D–F: adapted from Iversen and Rabbitt (2017).]