Figure 4.

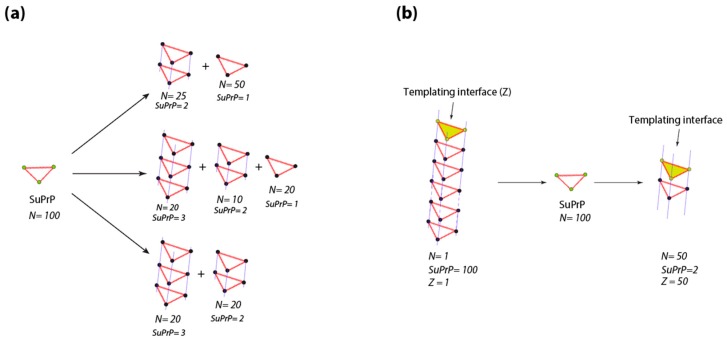
Relation between the number of templating interface and the detailed balance between PrP^Sc assemblies and suPrP. (a) According to the integer partition law and group representation theory ($p\left(N\right)=\frac{1}{4N\sqrt{3}}{e}^{\pi \sqrt{\frac{2N}{3}}}$, where N corresponds to the number of elements), 100 of molecules of suPrP can generate trough condensation 10⁸ possibility of PrP^Sc assemblies differing by size. As an illustration, three of them amongst the 10⁸ are presented. (b) A PrP^Sc assembly formed by the condensation of 100 suPrP presents only one templating interface (Z = 1). The existence of a detailed balance between PrP^Sc assemblies and suPrP makes that the PrP^Sc formed by 100 suPrP can rearrange into 50 PrP^Sc formed by the stacking of two suPrP, thus increasing the templating interface (Z = 50).