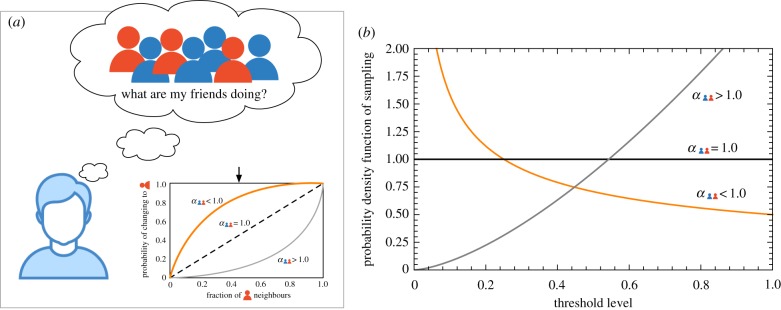
Figure 1.

(a) Graphical depiction of the complex contagion process under study. Individuals revise their state taking into consideration those of their friends, which, jointly with the complexity of the diffusing information, define the likelihood of an update (see equation (2.1)). (b) A popular approach in the literature is to consider threshold models, in which the probability of updating to a new state is one if the number of friends in such a state is above a specified threshold, being zero otherwise. Here we show that our model corresponds to considering a mean-field description of a population with a distribution of thresholds and holds similar dynamical properties. For instance, lower complexities are dynamically equivalent to scenarios with lower thresholds, while high complexities return a similar outcome as expected from high threshold processes. (Online version in colour.)