Figure 4.

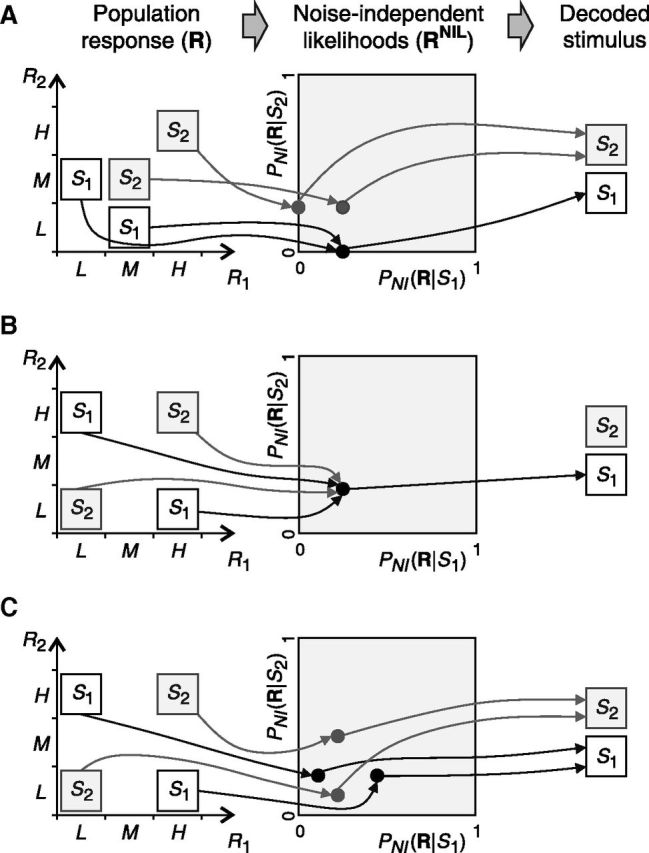
Examples of population activities decoded using the canonical NI decoder. The arrows show the transformation of population responses into vectors of NI likelihoods (R^NIL) induced by the NI assumption (left to middle) and optimal estimation algorithms (middle to right). In A, P(S₁) is set to 0.75, and P(M,L|S₁) and P(H,H|S₂) are both set to 0.5. In B and C, stimuli are equally likely and P(H,L|S₁) and P(H,H|S₂) are both set to 0.5 in B and to 0.66 in C. A, After the NI assumption, the distinction between responses elicited by different stimuli is preserved (middle panel). Therefore, ΔI_NI^NIL is zero and noise correlations are unimportant for decoding. B, After the NI assumption, all responses are identical. No information about the stimulus remains and noise correlations are crucial for decoding. C, However, whenever population responses are not equally likely given each stimulus, the NI assumption preserves all the encoded information and noise correlations are unimportant for decoding. The case shown in B, in which noise correlations are important, therefore constitutes an isolated example.