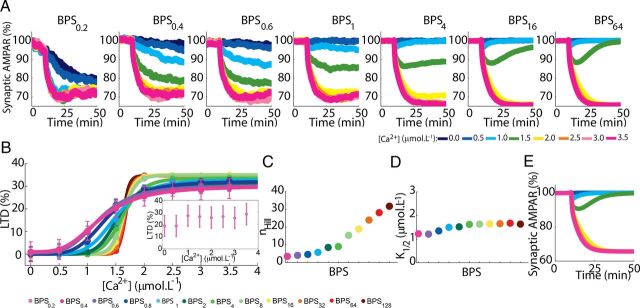
Figure 9.

Dependence of the macroscopic LTD responses on BPS. A, Macroscopic curves of LTD (means of 156 runs, Ca²⁺ pulses of 4 s applied at 10 min) showing the reduction in the variability and stability of responses when BPS is altered. Lowering BPS leads to a monostable system with spontaneous LTD while increasing it leads to a bistable system, similar to deterministic versions of the model (E). Stable intermediary mean levels of LTD, similar to those observed experimentally, are present only between BPS_0.6 to BPS₄. B, Sigmoidal relationship between the macroscopic LTD and peak [Ca²⁺] (pulses of 4 s) obtained using different BPSs. Data from 156 runs are plotted as mean ± SEM. Inset shows the responses of BPS_0.2 that could not be fitted with a sigmoid. C, D, n_Hill and K_1/2 for different BPSs. E, The same model was solved deterministically (using the Runge–Kutta method). In the absence of stochasticity the model shows a bistable behavior (see A for legend).