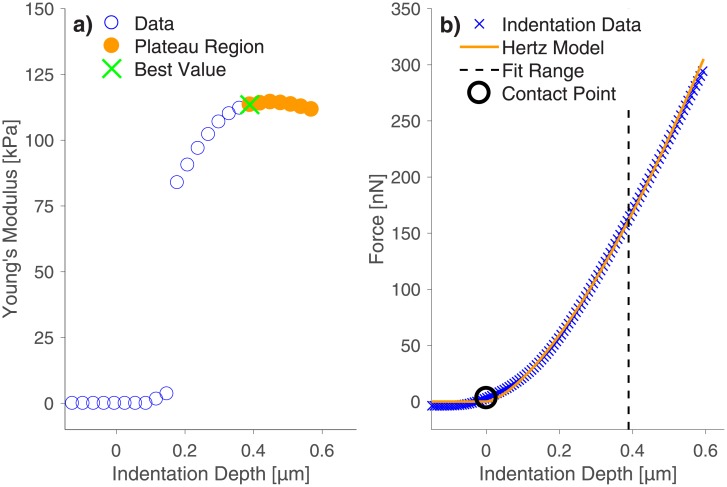
Fig 1. AFM indentation experiments on a thin PAAm sample.

a) Calculated Young’s moduli as a function of indentation depth show that a high enough indentation depth is necessary to reach a Young’s modulus value that is independent of indentation depth. This is important for the reliability and comparability of elasticity measurements. In order to enable the analysis of huge amounts of data (many measurements on different positions), we created an algorithm that detects the plateau region of the E-d curves automatically. The orange dots represent the plateau region of the Young’s modulus determined by our algorithm, whereas the green cross shows the Young’s modulus with the lowest error of the Hertzian fit detected by our algorithm. Furthermore, the used fit range and contact point are displayed in the corresponding force-distance curve (b).