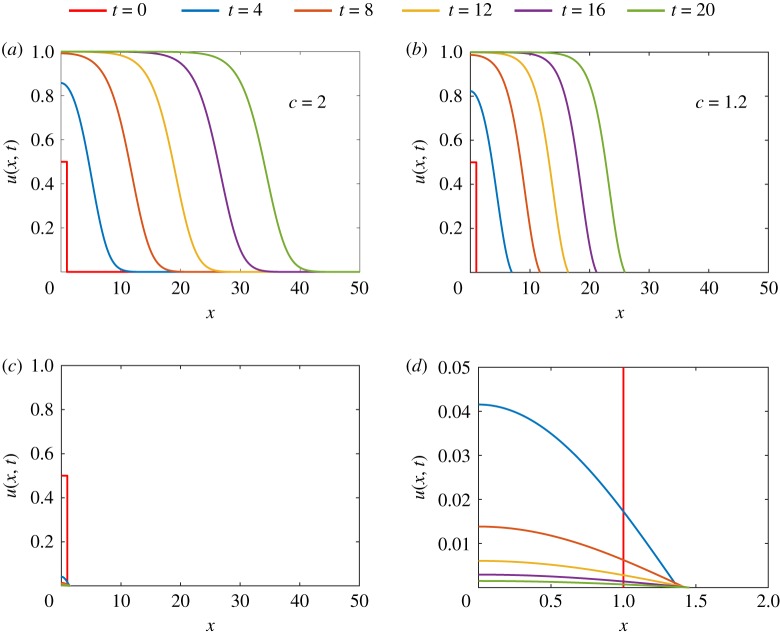
Figure 2.

Numerical solutions of the Fisher–KPP and the Fisher–Stefan models. (a) Numerical solutions of the Fisher–KPP equation evolving into a travelling wave solution with the minimum wave speed, c = 2. (b) Numerical solutions of the Fisher–Stefan model evolving into a travelling wave solution with c = 1.2. (c) Numerical solutions of the Fisher–Stefan model leading to extinction. (d) Magnified region of the solution in (c), for 0≤x≤2, to clearly show the dynamics of the extinction behaviour. For the Fisher–Stefan model, we set κ = 20 in (b) and κ = 0.45 in (c). Numerical solutions of the Fisher–Stefan model are obtained with Δξ = 1 × 10⁻⁴, whereas numerical solutions of the Fisher–KPP model are obtained with Δx = 1 × 10⁻⁴. For both, the Fisher–KPP and Fisher–Stefan models, we set Δt = 1 × 10⁻³ and ϵ = 1 × 10⁻⁸. For all results presented here, the initial condition is equation (2.4) with α = 0.5 and β = 1.