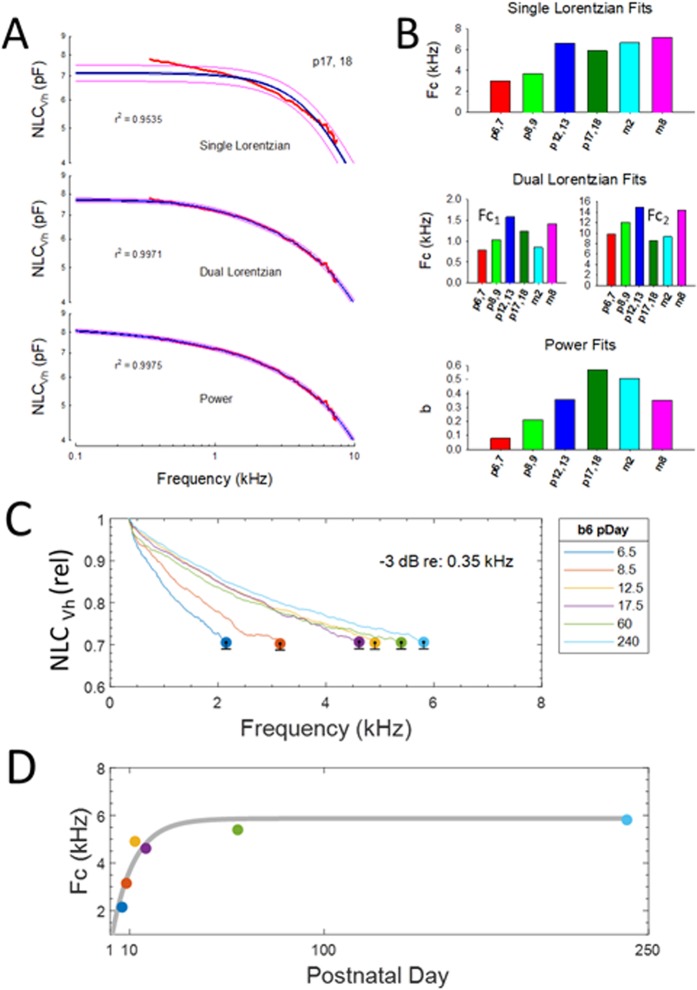
Figure 5.

Changes in frequency response of NLC during aging. (A) Three types of fits to mean NLC_Vh were performed to estimate frequency roll-off. Examples of the 3 fits for p17/18 OHCs are shown. Blue line is fit and red lines are 95% confidence predictions of the fits (done in Sigmaplot). The poorest fit is with a single Lorentzian, followed by dual Lorentzian and power fits (f in kHz). Nevertheless, each provides evidence for increases in frequency responsiveness during development. The <a> parameter, as all others, was not constrained, but was not age –dependent and similar for all fits [p6,7 - m8: −1.4055, −1.3548, −1.5980, −1.1960, −1.1552, −1.5031; mean +/− se −1.369 (0.07)]. The small se indicates little variability. (B) Bar plots of frequency cut-off parameters of the fits. (C) Another metric of frequency response roll-off was to determine the −3dB magnitude of NLC_Vh relative to 350 Hz values, denoted here with circles. It should be noted that the Fc’s simply reflect the relative roll-off during aging, and their absolute values will differ depending on the reference frequency. During the life span, Fc increases. The se indicates the variability at the cut-off frequencies. (D) The Fc data were fit to a power law function in Matlab (grey line; Fc = a.*(1-b.^pDay), where a = 5.867 and b = 0.9065; R² = 0.849), and indicates a stabilization near 6 kHz. P6,7 n = 7; p8,9 n = 7; p12,13 n = 6; p17,18 n = 8; 2 month n = 8; 8 month n = 9.