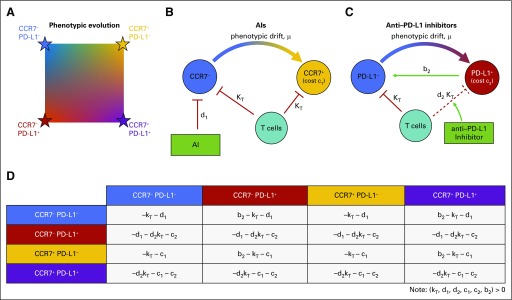
FIG 2.

Schematic of the mathematical model. (A) The tumor population consists of phenotypes varying on two axes: CCR7 (increasing from left to right) and PD-L1 (increasing from top to bottom). (B) Aromatase inhibitor (AI) therapy targets CCR7⁻ phenotypes with kill rate of d₁; these may evolve CCR7 expression due to selection. T cells kill both types at rate k_T. (C) Checkpoint inhibitor therapy boosts the immune response against tumor cells expressing PD-L1. In addition, PD-L1⁻ cells benefit from being near PD-L1⁺ cells, known as a “cheater” population. (D) The two-dimensional phenotypic space is modeled using a four-player game with subscript 1 indicating the CCR7 axis and subscript 2 indicating the PD-L1 axis. Killing terms (see red lines in 2B and 2C) for k_T, d₁, and d₂ are added to PD-L1⁻, CCR7⁻, and PD-L1⁺ rows, respectively. Cost terms (c₁, c₂) are added to CCR7⁺ and PD-L1⁺ rows, respectively. The cheater benefit, b₂, is added to PD-L1⁻ interactions (row) with PD-L1⁺ cells (column). Note that all costs (c₁, c₂, k_T, d₁, d₂) and benefits (b₂) in payoff matrix A are constrained to non-negative values.