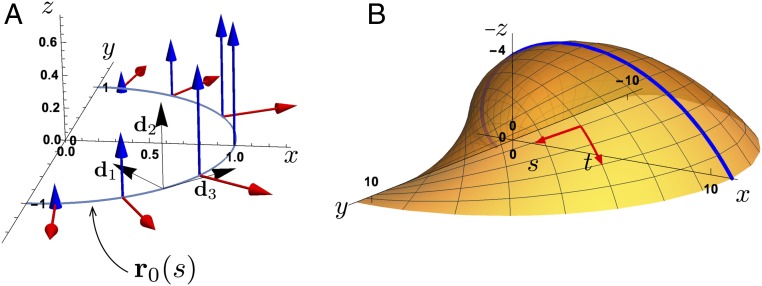
Fig. 2.

(A) The base geometry for bivalved shells is constructed via a locally defined growth velocity field defined on a base curve ${\mathbf{r}}_0\left(s\right)$ equipped with orthonormal basis $\left\{{\mathbf{d}}_1,{\mathbf{d}}_2,{\mathbf{d}}_3\right\}$. The growth consists of dilation (red arrows) and a coiling velocity in the binormal (${\mathbf{d}}_2$) direction with linear gradient (blue arrows) and hinge along the $y$ axis. (B) The resulting surface for one valve of the bivalve shell, with the $s$ and $t$ directions highlighted as well as the longitudinal midline (the curve $s=0$), which forms a logarithmic spiral.