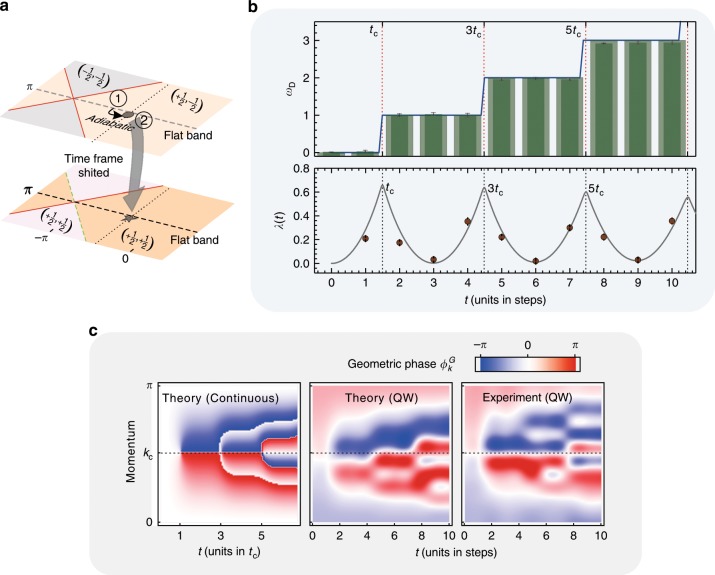
Fig. 5. Observation of the DQPT in a quench by shifting the time frame.

The strategy is presented in a in terms of the phase diagram. We initialize the system in a ground state of a given Hamiltonian H(θ₁, θ₂) with θ₁ = −π/3, θ₂ = 8.6π/9, which is located in the trivial phase. We adopt adiabatic evolution starting from the flat band again to prepare the initial state. With the time frame shifted, the winding of the eigenvectors of the given Hamiltonian changes its features, from zero to ±1. We show the DTOP in b and the PGP in c. The errors are estimated using numerical Monte Carlo simulations considering the counting noise.