Figure 2.

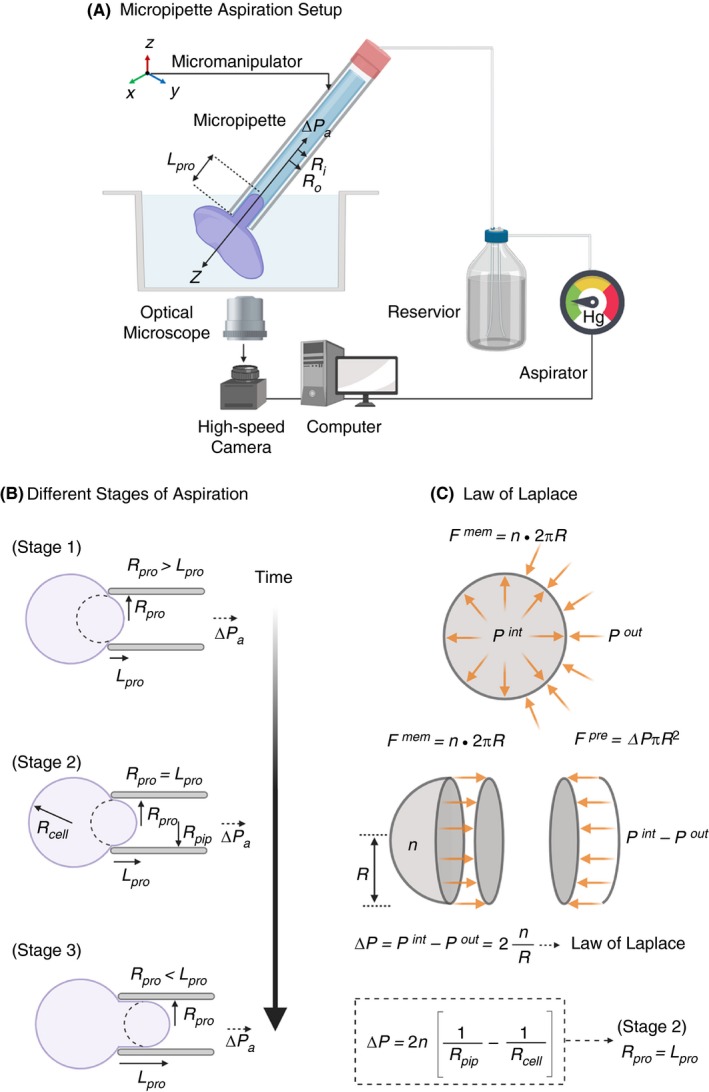
A, Schematic diagram of micropipette aspiration setup of a single resting platelet. The micropipette movement immersed in platelet suspension is controlled by a precision micromanipulator, ΔPa is the aspiration pressure applied parallel to the z‐axis through the aspirator, Ri and Ro‐inner and outer radius of the micropipette respectively and L _pro is the length of the protrusion of platelet body into the micropipette. Change in L _pro is recorded continuously with a high‐speed camera. B, Micropipette aspiration is a dynamic procedure with three distinct stages: In Stage I (initial), radius of protrusion R _pro > L _pro, the length of protrusion. During Stage II (critical) R _pro = L _pro and in Stage III (final) R _pro < L _pro. In Stage II when L _pro/R _pro = 1, the Law of Laplace can be applied to determine the surface tension n (SI units pN/μm). C, Simplified schematics of the Law of Laplace, which provides a relationship between the radius R of a membrane of a spherical cell subjected to the pressure difference ΔP = P ^int − P ^out, where P ^int and P ^out are the pressures inside and outside the cell, respectively. The membrane force F ^mem = n 2πR is the result of the surface tension n acting on the cell membrane along the circumference C = 2PR as shown in the free body diagram of a spherical cell cut in half. It is in equilibrium with the forces F ^pre resulting from the pressure difference ΔP acting on the cell area A = PR ². Combining these two we arrive at the Law of Laplace, which gives the relationship between cell wall and its curvature 1/R in terms with the surface tension n. Applying this to the critical Stage II during micropipette aspiration where R _pro = L _pro for radius of protrusion and that of cell we can effectively determination the surface tension n of the cell. (Figure modified from Gonzalez‐Bermudez et al.15)