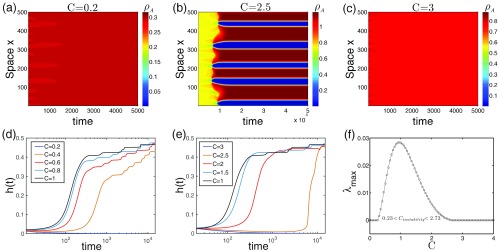
FIG. 3.

The impact of contagion interactions. Upper row: The spatiotemporal evolution of overall density of infection A (${\rho }_{{}_A}=I_A+I_{AB}$) for $C=0.2,2.5,3$, which shows that strong competition, e.g., $C=0.2$ in (a) or strong cooperation like $C=3$ in (c) actually inhibits patterns. (b) A case with a relatively strong interaction ($C=2.5$) that takes a long time to develop patterns. Lower row: $h\left(t\right)$ for a couple of interaction $C$ indicates that patterns are most likely to happen in the case of $C\simeq 1$ [(d) and (e)]; any deviation to a smaller or larger value will delay or just fail to have the formation process. (f) Eigenvalue analysis shows that the pattern appears within $0.23<C_{\mathrm{instability}}<2.73$, where ${\lambda }_{\max }>0$ and the peak is around $C=1$, in line with the observations here. Parameters: $R_0=2$, $D_S=10$, $D_I=1$. Random initial conditions are used in (a)–(e).