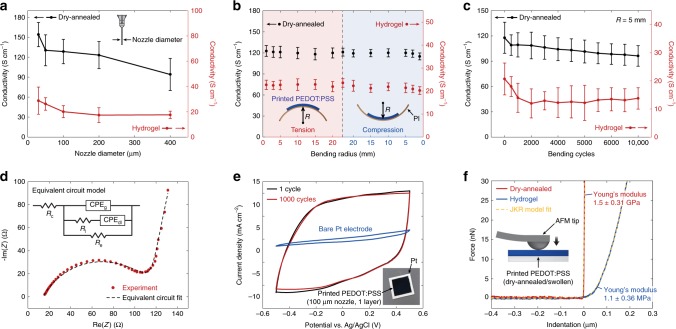
Fig. 3. Properties of 3D-printed conducting polymers.

a Conductivity as a function of nozzle diameter for 3D-printed conducting polymers in dry and hydrogel states. b Conductivity as a function of bending radius for 3D-printed conducting polymers in dry (17 µm, thickness) and hydrogel (78 µm, thickness) states. PI indicates polyimide. c Conductivity as a function of bending cycles for 3D-printed conducting polymers in dry (17 µm, thickness) and hydrogel (78 µm, thickness) states. d Nyquist plot obtained from the EIS characterization for a 3D-printed conducting polymer on Pt substrate (78 µm, thickness) overlaid with the plot predicted from the corresponding equivalent circuit model³⁸. In the equivalent circuit models, R_e represents electronic resistance, R_i represents ionic resistance, R_c represents the total ohmic resistance of the cell assembly, CPE_dl represents the double-layer constant phase element (CPE), whereas CPE_g represents the geometric CPE. CPE is used to account inhomogeneous or imperfect capacitance and are represented by the parameters Q and n where Q represents the peudocapacitance value and n represents the deviation from ideal capacitive behavior. The true capacitance C can be calculated from these parameters by using the relationship C = Qω_maxⁿ⁻¹, where ω_max is the frequency at which the imaginary component reaches a maximum³⁷. The fitted values for 3D-printed PEDOT:PSS are R_e = 107.1 Ω, R_i = 105.5 Ω, R_c = 14.07 Ω, Q_dl = 1.467 × 10⁻⁵ F sⁿ⁻¹, n_dl = 0.924, Q_g = 4.446 × 10⁻⁷ F sⁿ⁻¹, and n_dl = 0.647. e CV characterization for a 3D-printed conducting polymer on Pt substrate. f Nanoindentation characterizations for 3D-printed conducting polymers in dry and hydrogel states with the JKR model fits. Values in (a–c) represent the mean and the standard deviation (n = 5 per each testing conditions based on independently prepared samples and performed experiments).