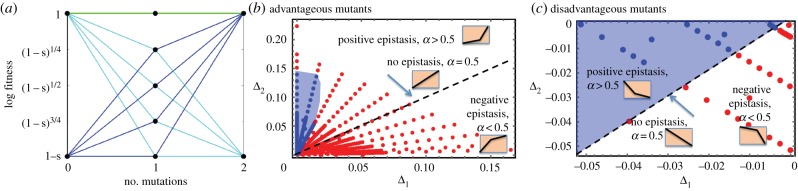
Figure 1.
Summary of different scenarios. (a) Examples of fitness landscapes used in the simulations neutral (green), disadvantageous (cyan) and advantageous (dark blue). Without epistasis the single-mutant fitness is given by f = (1 − s)1/2. For negative and positive epistasis examples in the figure, it is given by (1 − s)1/4 and (1 − s)3/4, respectively. For the extreme form of positive epistasis, single-mutant fitness is the same as that of wild-types, 1 − s. (b,c) Role of recombination for different fitness landscapes. The horizontal axis is Δ1 and the vertical axis is Δ2, which are the relative log fitness values of single and double mutants, respectively. Each of the dots corresponds to a particular fitness landscape. (b) Advantageous mutants: red dots correspond to runs in which recombination accelerated double-hit mutant invasion to 90%, while blue dots indicate that recombination slowed down invasion. The boundary between the regions represents where there are no significant results one way or another. (c) Disadvantageous mutants: red dots indicate that recombination increased the double mutant fraction at T = 105, while blue dots mean that recombination reduced the double mutant fraction. Blue shading marks the regions where recombination suppresses double-hit mutants. The dashed black line corresponds to the cases of no epistasis (α = 0.5) and separates the regions with positive epistasis (α > 0.5) and negative epistasis (α < 0.5). For both (b,c), we fixed the probability of free-virus transmission at 40% (β = 0.04); the rest of the parameters are as in figure 2. The determination of whether recombination suppressed or enhanced double-hit mutants was made by a statistical comparison of the averages over many runs, using the t-test.