Correlation between relative growth rate and specific leaf area requires associations of specific leaf area with nitrogen absorption rate of roots

Summary

• • Close correlations between specific leaf area (SLA) and relative growth rate (RGR) have been reported in many studies. However, theoretically, SLA by itself has small net positive effect on RGR because any increase in SLA inevitably causes a decrease in area‐based leaf nitrogen concentration (LNC_a), another RGR component. It was hypothesized that, for a correlation between SLA and RGR, SLA needs to be associated with specific nitrogen absorption rate of roots (SAR), which counteracts the negative effect of SLA on LNC_a.
• • Five trees and six herbs were grown under optimal conditions and relationships between SAR and RGR components were analyzed using a model based on balanced growth hypothesis.
• • SLA varied 1.9‐fold between species. Simulations predicted that, if SAR is not associated with SLA, this variation in SLA would cause a 47% decrease in LNC_a along the SLA gradient, leading to a marginal net positive effect on RGR. In reality, SAR was positively related to SLA, showing a 3.9‐fold variation, which largely compensated for the negative effect of SLA on LNC_a. Consequently, LNC_a values were almost constant across species and a positive SLA–RGR relationship was achieved.
• • These results highlight the importance of leaf–root interactions in understanding interspecific differences in RGR.

Introduction

Plant species vary widely in their relative growth rate (RGR), even when grown under uniform, close‐to‐optimal conditions. In recent decades, intensive attempts have been made to determine the physiological and morphological traits that cause inherent differences in RGR. The general approach is to break RGR down into three components, as follows:

RGR = SLA × NAR × LMR  (Eqn 1)

where SLA is the specific leaf area (leaf area per unit leaf mass), NAR is the net assimilation rate (rate of dry mass production per unit leaf area and time), and LMR is the leaf mass ratio (ratio of leaf mass to total dry mass). NAR may be further broken down into two components: leaf nitrogen productivity (LNP) and area‐based leaf nitrogen concentration (LNC_a), as follows:

RGR = SLA × LNP × LNC_a × LMR  (Eqn 2)

Among these variables, SLA has been shown to correlate with RGR fairly consistently and strongly (Poorter & Remkes, 1990; Lambers & Poorter, 1992; Cornelissen et al., 1996; Atkin et al., 1998; Poorter & Van der Werf, 1998; Reich et al., 1998a; Wright & Westoby, 2000; but see also Shipley, 2006).

However, SLA might have a less positive effect on RGR than one would conclude from these analyses because, according to the balanced growth hypothesis (Brower, 1962; Davidson, 1969; Hilbert, 1990; Garnier, 1991), any increase in SLA should inevitably cause a decrease in other components of RGR. The balanced growth hypothesis describes plant nitrogen concentration (PNC) as the ratio of the total nitrogen absorbed by a plant per unit time to the total biomass produced per unit time:

(Eqn 3)

where SAR is the specific absorption rate (amount of nitrogen absorbed per unit root mass and time) and RMR is the root mass ratio (ratio of root biomass to total plant biomass). Given that NAR is a product of LNP and LNC_a and that mass‐based leaf nitrogen concentration (LNC_m) is proportional to PNC (LNC_m=aPNC, where ‘a’ is a constant), Eqn 3 becomes:

Since LNC_a is a product of LNC_m and SLA⁻¹, this equation is further changed to:

Thus,

(Eqn 4)

Equation 4 predicts that any increase in SLA should cause a decrease in LNC_a if other variables remain constant. The decrease in LNC_a may be compensated if plants decrease their LMR/RMR (leaf : root ratio) with increasing SLA. In either case, however, an increase in SLA should be paid by a decrease in other RGR components, LNC_a or LMR, leading to a marginal net positive effect of SLA on RGR.

The reason that many previous studies nonetheless report a strong association between SLA and RGR could be that SLA correlates with other factors that have positive effects on LNC_a. If so, the decreases in LNC_a caused by SLA are compensated for, and an ‘apparent’ correlation between RGR and SLA may be established. From Eqn 4, the negative effect of SLA can be compensated if LNP or ‘a’ negatively, or SAR positively, correlates with SLA. However, LNP may generally positively correlate with SLA (Wright & Westoby, 2000), and nitrogen allocation between organs may not be systematically different between species (Osone & Tateno, 2005b). Thus, the most likely candidate appears to be SAR. In fact, Osone & Tateno (2005b) demonstrated, by means of a comparative experiment and a physiologically controlled experiment, that SAR positively affects leaf : root ratio, leaf nitrogen, and maximum photosynthesis rate. Furthermore, limited studies showed that SLA correlates positively with SAR across species, and the close associations between SAR and RGR reported in these studies also suggest a strong involvement of SAR in determining RGR (Poorter & Remkes, 1990; Garnier, 1991; Poorter et al., 1991; Reich et al., 1998b; Wright & Westoby, 2000). However, how SAR interacts with each of these leaf parameters and how much SAR contributes to RGR remain poorly understood.

The purpose of the present study was to examine the interaction between SAR and RGR components in determining RGR. We hypothesized that SAR is positively associated with SLA across species, and that SAR offsets the negative effects of SLA on LNC_a, and thereby allows a positive correlation between SLA and RGR. If these hypotheses are true, however, it is difficult to quantify the gross positive effects of SAR and gross negative effects of SLA on LNC_a from the observed pattern of LNC_a, because the observed patterns only represent the outcome after these effects of SAR and SLA have been offset. To estimate these opposing effects on LNC_a, we performed simulations based on the balanced growth hypothesis. We also investigated whether these hypotheses could apply to other datasets in the literature.

Materials and Methods

Plant materials

Five deciduous woody species and six herbs typical of open sunny habitats such as secondary forest, abandoned cropland, and roadsides were used in this study. The tree species comprised Prunus lannesiana var. speciosa Makino, Zelkova serrata (Thunb.), Morus bombycis Koidz., Salix integra Thunb. ex Murray, and S. gilgiata Seemen. The herbs comprised two perennial species, Polygonum cuspidatum Sieb. et Zucc. and Boehmeria nipononivea Koidz., and four annuals, Chenopodium album L., Bidens frondosa L., Achyranthes fauriei Lev. et Van, and Ipomoea nil (L.) Roth. Seeds were collected from individual populations in the Tochigi and Ibaraki prefectures, Japan. In the case of Z. serrata, seedlings with a cotyledon and two or three leaves were collected from a field at the Forest Products Research Institute in Ibaraki prefecture in late April 2005.

Growth conditions

The experiments were conducted in a naturally illuminated growth chamber with a temperature and humidity control unit at the Forestry and Forest Products Research Institute in Tsukuba, Japan (36°01′N, 140°25′E), between May and early July 2005. The tree species, which tend to grow slowly, were germinated in advance of the herbs in order to reduce the variation in initial seedling mass between the two groups. Seeds of all tree species except Salix spp. and Z. serrata were germinated on moist vermiculite or moist filter paper, and the seedlings with two leaves were transplanted individually into 1.6 l polyethylene pots filled with washed river sand. The collected Z. serrata seedlings were first transplanted onto moist vermiculite for 1 wk to allow the roots to recover from any damage or injury sustained at the time of collection and then transplanted individually into pots. Seeds of the herb species and Salix spp. were germinated directly in polyethylene pots filled with washed river sand then the seedlings were thinned to only one per pot. After transplanting, the plants were supplied with a nutrient solution consisting of 10 mm NH₄NO₃, 3 mm K₂HPO₄, 1 mm MgSO₄·7H₂O, 3 mm CaCl₂, 25 µm H₃BO₃, 2 µm MnSO₄·5H₂O, 2 µm ZnSO₄·7H₂O, 0.5 µm CuSO₄·5H₂O, 0.5 µm Na₂MoO₄·2H₂O, and 20 µm Fe‐EDTA (pH adjusted to 6.0 with 1 m HCl). Nutrient solution was given to allow the species to absorb nitrogen at their potential rates through the entire experimental period. Since the maximum nitrogen absorption rate reported in an earlier study was 0.1 g N g⁻¹ d⁻¹ for a herb species (Poorter et al., 1991), the nitrogen content of the pots was maintained well above a concentration that allowed absorption at this maximum. In the early stages of the experiments, each plant was given 500 ml of nutrient solution once a day, then later, when the plants were larger, twice a day to avoid nutrient depletion. Every 2 d, the pots were flushed with tap water to avoid salt accumulation.

The air temperature in the chamber was maintained between 22 and 26°C over each 24 h period to follow the daily time course of the photosynthetic photon flux density (PPFD). Relative humidity was maintained at 75% throughout. The seedlings were exposed to full sunlight at all times (the chamber was made of glass) and were randomly rearranged within the chamber every 2 d.

Harvesting

Because growth parameters vary with plant size, they should be compared among individuals of a similar size. To ensure plants of a similar size, woody species were harvested at 14 d intervals and herb species at 8 d intervals three times. The first harvest was performed on 8 June 2005 for woody species and 13 June 2005 for herbs. At each harvest, four to six individuals per species were sampled. The plants were divided into leaves, stems, and roots and leaf area was determined immediately using a flatbed scanner and image analysis software (Image J, http://rsb.info.nih.gov.IJ/). The dry mass of each plant part was determined after oven‐drying at 70°C for 3 d. The nitrogen content of each plant part was then determined using an automatic N/C analyzer (NC‐80; Shimadzu, Kyoto, Japan).

Data analysis

Growth components were calculated following the classical growth analysis method, in which changes in leaf area and biomass between two harvests are assumed to be exponential (Hunt, 1982). LNP was calculated as (log_e(X ₂) − log_e(X ₁))/(X ₂ –X ₁) × (Y ₁–Y ₂)/(t ₂–t ₁) where X _n is total leaf nitrogen, Y _n is plant biomass and t_n is time at the nth sampling. SAR was calculated analogously as X_n is root biomass and Y_n is total plant nitrogen at the nth sampling. For these calculations, the data obtained at the first and second harvests, at which the plant mass substantially overlapped among species (0.37 ± 0.05 g at the first harvest and 2.21 ± 0.28 g at the second), were used. Regression analyses were performed to examine the relationships between growth parameters using the statistical package SPSS (version 12.0 J; SPSS Japan Inc., Tokyo, Japan).

Comparison with other datasets

In order to make comparisons with earlier studies, data were collected from a series of experiments conducted by Poorter and co‐workers (Poorter & Remkes, 1990; Poorter et al., 1990, 1991) and Reich et al. (1998a,b) using DIGITIZEIT 1.5.7 (http://www.digitizeit.de; ShareIT! Inc., Cologne, Germany). LNC_a, leaf : root ratio and LNP, which were not presented in these studies, were calculated as the leaf nitrogen content per leaf mass divided by SLA, LMR divided by RMR, and NAR divided by LNC_a, respectively.

Using the pooled data collected from the present and previously mentioned studies, direct and indirect relationships between variables were analyzed through Path analyses using the statistical package Amos (version 6.0; SPSS Japan Inc., Tokyo, Japan). Before Path analysis, all data were ln‐transformed. Based on the balanced growth hypothesis, our path model assumed that SLA, LNP, leaf : root ratio and SAR affect LNC_a, and that the leaf : root ratio adjusts the balance between carbon inflow (SLA and LNP) and nitrogen inflow (SAR). The relationships between LNP, SLA, and SAR were set as covariations, that is, with no direct effects between these variables. However, it is conceivable that SLA, in part, directly affects LNP, since it is mostly determined by lamina thickness and the density of the leaf tissues, which affect the net photosynthetic rate per unit leaf nitrogen by influencing the degree of attenuation of photons passing through and the rate of CO₂ diffusion in the leaves (Hikosaka, et al., 1998; Poorter & Evans, 1998). However, anatomical factors are not the only factors affecting photosynthetic nitrogen‐use efficiency. Hikosaka et al. (1998) showed that differ‐ences in allocation of nitrogen to ribulose‐1,5‐bisphosphate carboxylase, a key enzyme of photosynthesis, and specific activity of ribulose‐1,5‐bisphosphate carboxylase also affect photosynthetic nitrogen‐use efficiency. Thus, we assumed that SLA and LNP would covary with each other so as to follow the ‘plant trait syndrome’, that is, multiple plant traits having evolved in a coordinated manner showing a specific combination between species (Reich et al., 1992; Grime, 2001).

Results

Correlations between RGR, leaf and root parameters

Across all species, RGR varied 2.5‐fold (Fig. 1). SLA showed a strong positive relationship with RGR (r ² = 0.55, P = 0.009, Fig. 1a). NAR was also positively correlated with RGR (r ² = 0.43, P = 0.017, Fig. 1b), which was largely ascribed to the close relationship between LNP and RGR (r ² = 0.67, P < 0.001, Fig. 1c). LNC_a, the other component of NAR, was not significantly correlated with RGR (r ² = 0.004, P = 0.86, Fig. 1d). LMR tended to increase with RGR, but the relationship was not statistically significant (r ² = 0.25, P = 0.069, Fig. 1e). Nitrogen absorption rates per unit root mass (SAR) varied 3.9‐fold among the species and exhibited a close relationship with RGR (r ² = 0.91, P < 0.001, Fig. 1f).

Figure 1.

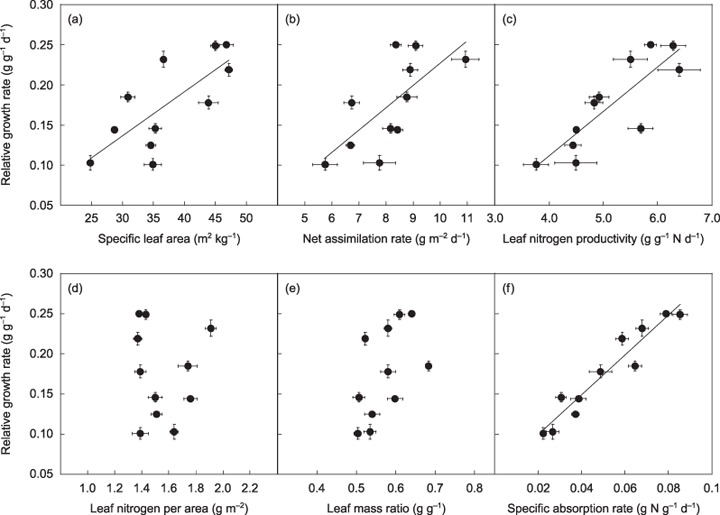
Relationships between relative growth rate and specific leaf area (a), net assimilation rate (b), leaf nitrogen productivity (c), leaf nitrogen per area (d), leaf mass ratio (e) and specific absorption rate (f). Bold lines represent regression lines for statistically significant relationships. Regression relationships: (a) y = 0.005x − 0.028 (r ² = 0.55, P = 0.009); (b) y = 0.028x − 0.050 (r ² = 0.43, P = 0.017); (c) y = 0.055x − 0.11 (r ² = 0.67, P < 0.001); (d) y = 0.021x + 0.14 (r ² = 0.004, P = 0.86); (e) y = 0.55x − 0.14 (r ² = 0.25, P = 0.069); (f) y = 2.48x + 0.05 (r ² = 0.91, P < 0.001). Bars represent ± SE of the mean.

Interaction between nitrogen absorption rates and the growth components

Specific leaf area varied 1.9‐fold between species (Fig. 1a). The effect this variation had on LNC_a was simulated (simulation 1, Fig. 2). In this simulation, LNC_as were calculated using Eqn 4 by varying SLA as observed for species (24.8–47.2 m² kg⁻¹), while other parameters in the equation were kept constant between species using the values obtained from Z. serrata, the lowest SLA species (SAR, 0.0266 g N g⁻¹ d⁻¹; LNP, 4.72 g g⁻¹ N d⁻¹; leaf : root ratio, 4.15; a, 1.35). By varying only SLA, this simulation showed the sole effect of SLA on LNC_a. The calculated LNC_a showed a 47% decrease along the SLA gradient, which represents the negative effects of SLA on LNC_a (Fig. 2a, broken line). In contrast to the simulation, the measured LNC_a showed only a small decrease with SLA (Fig. 2a, closed circles). This inconsistency suggests that there are one or more factors compensating for the negative effect of SLA on LNC_a preventing LNC_a of larger SLA species from decreasing.

Figure 2.

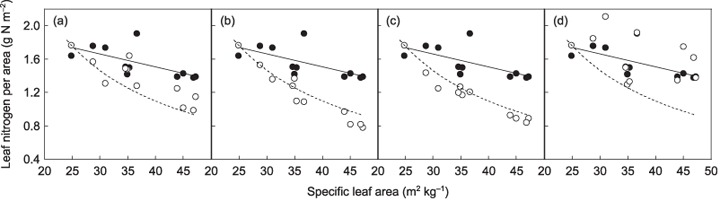
Simulations based on the balanced growth hypothesis. (a) Measured and calculated area‐based leaf nitrogen concentration (LNC_a) in simulations 1 and 2. Simulation 1 estimated the effect of specific leaf area (SLA) on LNC_a, and simulation 2 estimated the coupled effects of SLA and leaf : root ratio. Closed circles, measured LNC_a; open circles, calculated LNC_a in simulation 2. A broken line represents the result of simulation 1. A solid line represents the regression relationship between measured LNC_a and SLA, y = −0.016x + 2.14 (r ² = 0.42, P = 0.032). (b) Calculated LNC_a in simulation 3 that estimated the coupled effects of SLA and leaf nitrogen productivity (LNP). Open circles, calculated LNC_a in simulation 3. Other symbols and lines were as in (a). (c) Calculated LNC_a in simulation 4 that estimated the coupled effects of SLA and a. Open circles, calculated LNC_a in simulation 4. Other symbols and lines were as in (a). (d) Calculated LNC_a in simulation 5 that estimated the coupled effects of SLA and specific nitrogen absorption rate of roots (SAR). Open circles, calculated LNC_a in simulation 5. Other symbols and lines were as in (a).

Figure 3 shows the relationships between SLA and the other parameters in Eqn 4. The leaf : root ratio decreased slightly with SLA (Fig. 3a). Although the trend was not statistically significant, this may to some extent compensate for the negative effect of SLA. To estimate how much of an effect this might have, we calculated species LNC_a values using Eqn 4, assigning measured species values to SLA and the leaf : root ratio, while fixing other parameters with the values obtained from Z. serrata between species (simulation 2). LNC_a values calculated in this simulation (Fig. 2a, open circles) were generally larger than those in simulation 1, but still smaller than the measured LNC_a values. This suggests that the leaf : root ratio partly compensated for the negative effect of SLA on LNC_a, but there should be factors also affecting LNC_a positively. LNP increased with SLA (Fig. 3b). Since an increase in LNP negatively affects LNC_a (Eqn 4), this should not compensate for the negative effect of SLA. In a similar manner to simulation 2, the coupled effects of SLA and LNP on LNC_a values were calculated assigning measured species values to SLA and LNP in Eqn 4 while fixing other parameters (simulation 3). The calculated LNC_a values showed only a slight decrease compared with simulation 1 (Fig. 2b), suggesting that the negative effect of LNP was small, even though LNP showed the same degree of variation as SLA (Fig. 1a,c). The coefficient of the relationship between mass‐based leaf nitrogen concentration and plant nitrogen concentration, a, was almost constant across species (Fig. 3c), and therefore had little effect on LNC_a (Fig. 2c, simulation 4). Finally, SAR showed a large increase with SLA (Fig. 3d). LNC_a values calculated using Eqn 4 by assigning observed values to SLA and SAR with all other parameters kept constant across species were much larger than those in simulation 1 and close to the measured LNC_a values (Fig. 2d, simulation 5). This suggests that the negative effect of SLA on LNC_a was largely compensated for by SAR.

Figure 3.

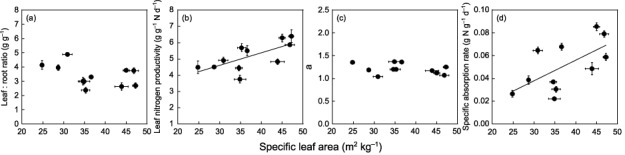
Relationships between specific leaf area and parameters of balanced growth hypothesis, leaf : root ratio (a), leaf nitrogen productivity (b), a (c) and specific absorption rate (d). Bold lines represent regression lines for statistically significant relationships. Regression relationships: (a) y = −0.034x + 4.52 (r ² = 0.12, P = 0.29); (b) y = 0.078x + 2.26 (r ² = 0.43, P = 0.017); (c) y = −0.005x + 1.39 (r ² = 0.11, P = 0.33); (d) y = 0.002x − 0.17 (r ² = 0.41, P = 0.035). Bars represent ± SE of the mean.

If SAR does not correlate with SLA, what would be the relationship between RGR and components of RGR? We calculated species LNC_a values using Eqn 4, assuming SAR to be constant across species and taking other parameters as measured for each species, and then calculated RGR with Eqn 2, using the obtained LNC_a. Because of the smaller LNC_a of species with a larger SLA, the calculated RGR increased only marginally with increases in SLA (Fig. 4a). Although LNP by itself had a less negative effect on LNC_a (Fig. 2b), the slope of RGR on LNP was also reduced because of the correlation with SLA (Fig. 4b). These results indicate that for there to be a positive correlation between RGR and SLA there must also be a positive relationship between SLA and SAR.

Figure 4.

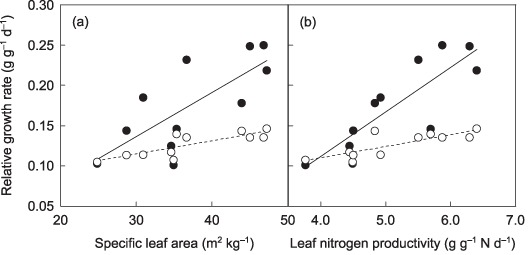
Simulated relationships between relative growth rate and specific leaf area (a), and relative growth rate and leaf nitrogen productivity (b). Closed circles, measured values; open circles, simulated values. For simulated relative growth rate (RGR) values, area‐based leaf nitrogen concentration (LNC_a) was first calculated using Eqn 4, assuming that parameters other than specific nitrogen absorption rate of roots (SAR) were constant across species (assuming that there was no positive effect of SAR); RGRs were then calculated with Eqn 2, assigning these LNC_a values. Regression relationships: (a) y = 0.005x − 0.028 (r ² = 0.55, P = 0.009) for measured values, y = 0.002x + 0.069 (r ² = 0.70, P = 0.001) for calculated values; (b) y = 0.028x − 0.050 (r ² = 0.43, P = 0.017) for measured values, y = 0.013x + 0.060 (r ² = 0.60, P = 0.003) for calculated values.

The interactions between SLA and SAR in determining LNC_a can be also shown on a graph where species are grouped by SLA (group 1, species with SLA < 30 m² kg⁻¹; group 2, species with SLA ≥ 30 m² kg⁻¹ and SLA ≤ 36 m² kg⁻¹; and group 3, species with SLA > 36 m² kg⁻¹; Fig. 5a). Across all species, LNC_a showed no significant relationship with SAR but instead showed convergence. However, within the five species with similar SLA (SLA between 30 and 36 m² kg⁻¹, closed circles), LNC_a showed a strong positive relationship with SAR (r² = 0.89, P = 0.001). As the variation in SLA was small among these species, this increase in LNC_a represents the positive effects of SAR on LNC_a, excluding the effects of SLA. On the other hand, a between‐group comparison showed the effects of SLA on LNC_a excluding the effects of SAR. At the same SAR, species with smaller SLA (open squares) generally showed a larger LNC_a than the five species described (located above the regression line of the five species), while those with larger SLA (open circles) showed a smaller LNC_a (located below the regression line of the five species), indicating that SLA negatively affected LNC_a. The apparent convergence of LNC_a when all species were included was thus a result of these opposing effects of SLA and SAR counteracting each other. Similar patterns were observed for the leaf : root ratio when grouping species by SLA (Fig. 5b); at a similar SAR, the leaf : root ratio was smaller in larger SLA species, while at a similar SLA, it was larger in larger‐SAR species. This suggests that the leaf : root ratio was also affected by SLA and SAR in a coordinated manner.

Figure 5.

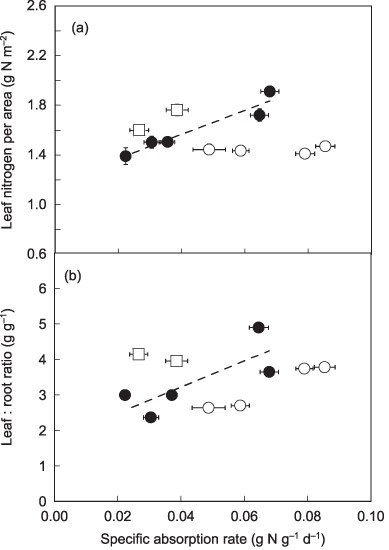
Leaf nitrogen per area (a) and leaf : root ratio (b) grouped by specific leaf area (SLA). Squares, species with SLA < 30; closed circles, species with SLA ≥ 30 and SLA ≤ 36; open circles, species with SLA > 36. Broken lines represent regression lines of the five species with intermediate SLA. Regression relationships: (a), y = 0.73x + 1.53 (r ² = 0.09, P = 0.78) for all species included, y = 9.67x + 1.18 (r ² = 0.89, P = 0.010), for species with SLA between 30 and 36; (b) y = 15.4x + 2.5 (r ² = 0.19, P = 0.16) for all species included, y = 44.6x + 1.5 (r ² = 0.67, P = 0.056) for five species with SLA between 30 and 36. Bars represent ± SE of the mean.

Comparison with other datasets

Figure 6 shows the data collected from studies by Poorter and co‐workers (Poorter & Remkes, 1990; Poorter et al., 1990, 1991) and by Reich et al. (1998a,b) together with the present data. Note that the parameters were plotted against SAR so as to contrast parameters that affect LNC_a negatively on the y‐axis with SAR, which affects LNC_a positively, on the x‐axis. As in the present study, SLA and LNP were strongly correlated with SAR in these earlier studies (Fig. 6a,b), although LNP showed distinct between‐study differences. The leaf : root ratio increased with SAR slightly in Poorter's dataset, but showed no consistent relationship in Reich's dataset (Fig. 6c). In Poorter's study, LNC_a decreased with SAR only slightly, suggesting that the negative effect on LNC_a was largely compensated for by SAR, as in the present study (Fig. 6d). On the other hand, in Reich's study, LNC_a decreased with SAR more greatly. At first glance, this was different from the present result, but in fact did not contradict the balanced growth hypothesis. In Reich's dataset, slopes of SLA and LNP on SAR were larger than those in the present study and Poorter's study, indicating that the negative effects of SLA and LNP on LNC_a were more dominant over the positive effects of SAR on LNC_a than in these other studies. Therefore, if the balanced growth hypothesis is true, LNC_a should decrease more steeply than the other datasets. Equation 4 showed that in Reich's dataset, where 3.6‐ and 3.4‐fold variations in SLA and LNP were associated with a 7.6‐fold variation in SAR, compensation of the negative effects of SLA and LNP by SAR should be 40% (LNC_a should decrease by 60% with increasing SAR). This is almost in accordance with the observed 64% decrease in LNC_a in the dataset, suggesting that the behavior of LNC_a followed the balanced growth hypothesis. As a result of the combined behaviors of LNC_a and LNP on SAR, NAR increased with SAR in the present and Reich's study and showed no consistent pattern with SAR in Poorter's study (Fig. 6e).

Figure 6.

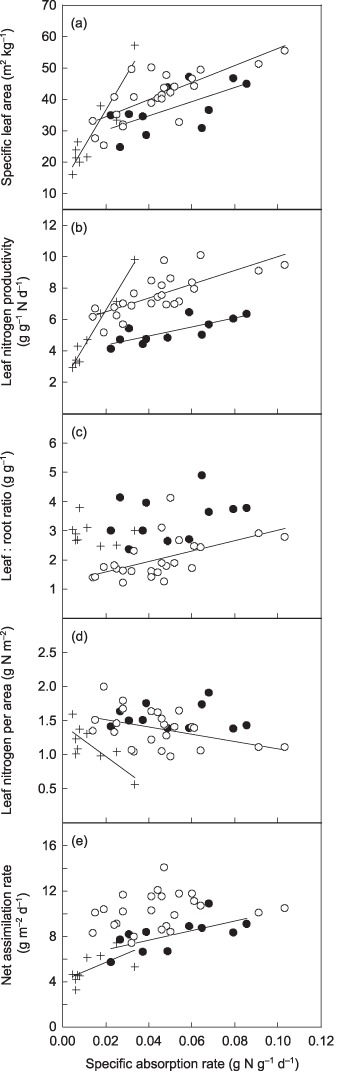
Interaction between leaf and root properties in the present study and in the two previous studies. Closed circles, data from the present study; open circles, data collected from studies by Poorter and co‐workers (Poorter & Remkes, 1990; Poorter et al., 1990, 1991); +, data collected from studies by Reich et al. (1998a,b). Bold lines represent regression lines for statistically significant relationships. Regression relationships – present study: specific leaf area (SLA), y = 224x + 26 (r ² = 0.34, P = 0.035); leaf nitrogen productivity (LNP), y = 28.9x + 3.7 (r ² = 0.49, P = 0.01); leaf : root ratio, y = 15.4x + 2.5 (r ² = 0.12, P = 0.16); leaf nitrogen per area (LNC_a), y = 0.028x + 1.55 (r ² = 0, P = 0.92); net assimilation rate (NAR), y = 42.2x + 6.0 (r ² = 0.36, P = 0.031); Poorter's study: SLA, y = 274x + 29 (r ² = 0.55, P < 0.001); LNP, y = 43.7x + 8.3 (r ² = 0.54, P < 0.001); leaf : root ratio, y = 17.7x + 1.2 (r ² = 0.27, P = 0.006); LNC_a, y = −5.32x + 1.62 (r ² = 0.15, P = 0.037); NAR, y = 18.7x + 9.4 (r ² = 0.023, P = 0.23); Reich's study: SLA, y = 1159x + 13 (r ² = 0.82, P < 0.001); LNP, y = 227x + 2.6 (r ² = 0.70, P < 0.001); leaf : root ratio, y = −9.64x + 3.002 (r ² = 0.058, P = 0.53); LNC_a, y = −22.8x + 1.4 (r ² = 0.56, P = 0.013); NAR, y = 79.1x + 4.1 (r ² = 0.31, P = 0.05).

To further confirm whether these correlations really reflect the hypothetical causalities, we performed path analyses (Fig. 7). The three datasets were pooled, excluding those for grass species in Poorter's study as they have a specifically smaller leaf : root ratio than the other species when ln‐transformed for the analyses; this gave a total of 33 species. The path model that was derived was not significantly rejected (χ² = 2.5, 1 df, P = 0.11). Further, each of the path coefficients predicted by the model was significantly different from zero (P < 0.05) and all signs of the path coefficients were in the predicted direction except for that from LNP to the leaf : root ratio. The lack of a significant relationship between LNP and the leaf : root ratio was caused by the relatively large between‐study differences in the two variables, which might reflect the differences in experimental conditions between studies. When the path was removed, the fit to data was improved (χ² = 4.0, 2 df, P = 0.14).

Figure 7.

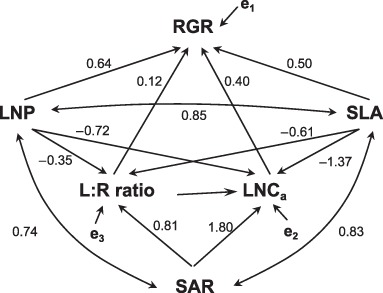
 A hypothetical path model for leaf and root properties. Single‐headed arrows represent direct relationship or causality. Double‐headed arrows represent indirect relationship or covariation. RGR, relative growth rate; SLA, specific leaf area; LNP, leaf nitrogen productivity; LNC_a, leaf nitrogen per area; L : R ratio, leaf : root ratio; SAR, specific absorption rate. e represents an error term. Numbers beside each arrow represent standardized path coefficients.

Discussion

Leaf and root properties and their interaction

Of the RGR components, SLA and LNP were more closely correlated with the variation in RGR than were LNC_a and LMR (Fig. 1). The strong correlations between RGR and SLA are in accordance with the findings of previous studies (Poorter & Remkes, 1990; Lambers & Poorter, 1992; Cornelissen et al., 1996; Atkin et al., 1998; Poorter & Van der Werf, 1998; Reich et al., 1998a; Wright & Westoby, 2000), and the correlations between RGR and LNP are also widely recognized (Garnier & Vancaeyzeele, 1994; Atkin et al., 1998; Wright & Westoby, 2000; Warren & Adams, 2005).

The present study suggests, however, that these correlations would not hold without an association between SLA and SAR, which compensates for the negative effect of SLA on LNC_a. Simulations predicted that, if SAR was not correlated with SLA, the 1.9‐fold variation in SLA observed in the present study would cause a 47% decrease in LNC_a along the SLA gradient (Fig. 2a), and thus, the SLA showed only a small increase with RGR (Fig. 4a). According to the balanced growth hypothesis (Eqn 4), to fully compensate for the negative effect of SLA (which varied 1.9‐fold between species) on LNC_a, SAR or leaf : root ratio should vary at least 3.6 (=1.9²)‐fold, being positively correlated with SLA, or a or LNP should vary 3.6‐ fold, being negatively correlated with SLA. In the present study, 3.9‐fold variation in SAR was associated with SLA, which was just enough to compensate for the negative effect of SLA resulting in a minimal decrease in LNC_a with increasing SLA (Fig. 2). Negative relationships between SLA and LNC_a (or NAR) were previously indicated in earlier studies (Poorter & Remkes, 1990; Biere, 1996; McKenna & Shipley, 1999; Wright & Westoby, 2000; Shipley, 2002). However, to our knowledge, this is the first paper to quantify the negative effect of SLA on LNC_a and show that a positive correlation between RGR and SLA requires a positive relationship between SLA and SAR.

When species were grouped by SLA, the leaf : root ratio showed clear patterns depending on SLA and SAR – an increase with SAR between species with similar SLA and a decrease with SLA between species with similar SAR values (Fig. 5b). Given that a larger SLA increases carbon inflow and a larger SAR increases nitrogen inflow to plants, these responses are analogous to the phenotypic response of the leaf : root ratio to changes in the external environment. In general, plants increase their leaf : root ratio in response to factors that increase nitrogen inflow relative to carbon inflow such as increased soil nitrogen availability, and decrease the ratio in response to factors that decrease nitrogen inflow relative to carbon inflow, such as increased light intensity (Kachi & Rorison, 1989; Olff, 1992; Poorter, 1999; Osone & Tateno, 2005a). These phenotypic responses are adaptive, allowing plants to compensate for relatively growth‐limiting resources and maintain high growth rates under a wide range of environmental conditions (Ågren & Ingestad, 1987; Hirose, 1987; Kachi & Rorison, 1989; Ishizaki et al., 2003; Osone & Tateno, 2005a). Similar to this phenotypic response, a plant species with a specific combination of SLA and SAR may adjust its biomass allocation to increase either the carbon or nitrogen inflow, depending on which is more growth‐limiting for the species. However, these trends were masked when all species were included in the comparisons, because the covariation of SLA and SAR caused counteraction of these confronting effects (Fig. 5b). This might be why the leaf : root ratio or LMR generally does not show a consistent pattern between species in comparative growth analysis (reviewed by Aerts & Chapin, 2000; Poorter & Nagel, 2000).

The simulations indicated that the negative effects of SLA on LNC_a were so large that they would cause only marginal net positive effects on RGR (Fig. 4a). This may give the impression that having larger SLA is of little advantage in increasing RGR. However, in fact, our calculations may have overestimated the negative effects of SLA and LNP on LNC_a and LMR because we did not consider a functional relationship between LNC_a and LNP. Since NAR is generally a curvilinear function of LNC_a, with the x‐intercept larger than zero (Hirose, 1986; Van der Werf et al., 1993; Osone & Tateno, 2005a,b), LNP is maximized at LNC_a when the tangent from the origin touches the NAR–LNC_a curve, and is decreased with LNC_a moving away from the point. If this relationship were included, the decreases in LNC_a of larger SLA species would be less than what was actually calculated, because the concomitant decreases in LNP should alleviate further decreases in LNC_a (see Eqn 4).

Theoretically, species differences in the nitrogen absorption rate per root mass can be caused by both differences in morphological (e.g. root surface area or root length per root mass) and physiological properties (e.g. numbers of channels on the root surface) of roots. The correlation between SAR and specific root length (root length per root mass) was reported in Reich et al. (1998b) and Osone & Tateno (2005b). On the other hand, Reich et al. (1998b) showed there were also substantial differences in area‐based nitrogen absorption rate (nitrogen absorbed per root surface area per unit time), suggesting an involvement of root physiology to species differences in SAR. In the present study, SRL varied approx. fivefold between species and positively related with SAR (r ² = 0.41, unpublished data), but the area‐based nitrogen absorption rate differed only 1.5‐fold between species. Thus, species differences in SAR may be largely caused by species differences in root morphology in the present study.

Generality of the relationships

The datasets from earlier studies (Poorter & Remkes, 1990; Poorter et al., 1990, 1991; Reich et al., 1998a,b) also showed positive SLA–SAR and LNP–SAR correlations (Fig. 6a,b). Consistent with the present study, the negative effects of SLA and LNP on LNC_a were largely compensated for by SAR in Poorter's dataset (Fig. 6d). However, in Reich's study, the negative effects were only compensated for by 36% (a 64% decrease in LNC_a, Fig. 6d). This lower degree of compensation might be caused by differences in the experimental conditions. In Reich's study, plants were given nutrient solution with a nitrogen concentration almost half that of the present study once a day, compared with twice a day in the present study. Therefore, species with higher carbon gain (i.e. higher SLA and LNP) might experience nitrogen depletion as they grow and absorb nitrogen at a lower rate than their potential, while other species might absorb nitrogen near their potential. This should result in a relatively small SAR to SLA (a steep SLA–SAR slope), and thus a decreased LNC_a in larger SLA species. From these results, we tentatively conclude that the covariation of SAR with SLA and LNP is a general trend, but that the degree of compensation by SAR may differ between datasets or experimental conditions. To generalize what experimental conditions causes partial or full compensation requires further studies.

There were distinct differences in LNP and the leaf : root ratio between the present and Poorter's study (Poorter & Remkes, 1990; Poorter et al., 1990, 1991); LNP was smaller and the leaf : root ratio was larger in the present study (Fig. 6b,c). This might be because of the difference in light conditions. In the present study, where plants were grown in a naturally illuminated growth chamber, they gradually closed their stomata from early afternoon when the light intensity was maximized (1700 µmol m⁻² s⁻¹) and accordingly the photosynthetic rate decreased. On the other hand, in Poorter's study, where plants were grown under a constant weaker photon flux density of 315 µmol m⁻² s⁻¹ under 14‐h‐day period, plants were possibly able to photosynthesize at a constant rate during the daytime. This might have caused a larger daily assimilation (NAR), and thus LNP, in Poorter's study than in the present study (Fig. 6b,e), although the average light intensity during the daytime was lower in the former. When carbon inflow is limiting, plants generally increase leaf : root ratio to compensate for the small carbon inflow (Hirose, 1987; Hilbert et al., 1991). Thus, the larger leaf : root ratio in the present study might be a response to the smaller daily assimilation (Fig. 6c). It is notable, however, that the LNC_a–SAR relationships were still conservative between the two studies (Fig. 6d), despite the large difference in LNP. These results suggest that the leaf and root physiological properties and biomass allocation are strictly coordinated between species.

Implications for the determinant of RGR

Despite broad agreement on the relationship between RGR and SLA, no consensus has been reached with regard to the relationships between RGR and NAR, and RGR and LMR, in studies of comparative growth analysis (reviewed by Poorter & Van der Werf, 1998). A possible explanation for the inconsistency is the differences in ambient light adopted in different studies (Poorter, 1999; Ryser & Wahl, 2001; Shipley, 2002, 2006; see also Medek et al., 2007). In an experiment where plants experience a weak ambient light at which photosynthesis is not light‐saturated, the species difference in NAR is small, and thus SLA remains the main determinant of RGR. Conversely, in an experiment where higher ambient light is adopted, species differences in NAR are highlighted, and thus NAR will become the main determinant of RGR. Shipley (2006) provided strong support for this contention by meta‐analysis, but also pointed out that substantial inconsistencies between studies still remain that cannot be accounted for by differences in light conditions.

The present study may provide a supplementary explanation for this issue. From our hypotheses, the pattern of species LNC_a in a dataset depends on the net effects of parameters that affect LNC_a both positively (SAR) and negatively (SLA, LNP). Therefore, theoretically, LNC_a could either decrease or increase independently with LNP between species. Since NAR is a product of LNC_a and LNP, these inconsistent behaviors of LNC_a and LNP may interfere with a consistent pattern of NAR between studies. In a dataset where LNC_a and LNP are negatively related, a positive relationship between LNP and RGR is always offset to a certain extent by the negative relationship between LNC_a and RGR. In this case, the extent of the relationship between RGR and NAR will be determined by the relative strength of the relationships between LNP and RGR, and between LNC_a and RGR in the dataset. The datasets of Poorter and Reich in Fig. 6 provide good examples of this. In the two datasets, the relationships between SAR and other parameters presented in Fig. 6 can be equated by the relationships between RGR and the parameters, since SAR was strongly related to RGR (P < 0.001). In Reich's study, LNP increased and LNC_a decreased with SAR (and thus RGR), and LNP showed a much steeper increase with SAR than the decrease in LNC_a with SAR (Figs 6b,d). Consequently, NAR, the product of LNP and LNC_a, showed a positive relationship with SAR (and thus RGR) (Fig. 6e). In Poorter's dataset, LNP and LNC_a also showed inverse trends with SAR, but the increase in LNP balanced the decrease in LNC_a (Fig. 6b,d). As a result, NAR was almost constant with SAR (and thus RGR) (Fig. 6e). In a dataset in which LNC_a does not show a distinct converse trend with LNP, without such countervailing effects between LNC_a and LNP, a positive relationship between NAR and RGR might exist. An example of this is provided by the present study. In the present study, LNC_a was almost constant to RGR (Fig. 1d), while LNP increased with RGR (Fig. 1c), which results in NAR being positively related to RGR (Fig. 1b). Similarly, Wright & Westoby (2000) suggested that the negative correlation between LNP and LNC_a and the different degrees of correlation between studies could cause the lack of a general pattern in the RGR–NAR relationship between studies.