FIG. 3. A cell as a viscous interior surrounded by an actomyosin cortex.

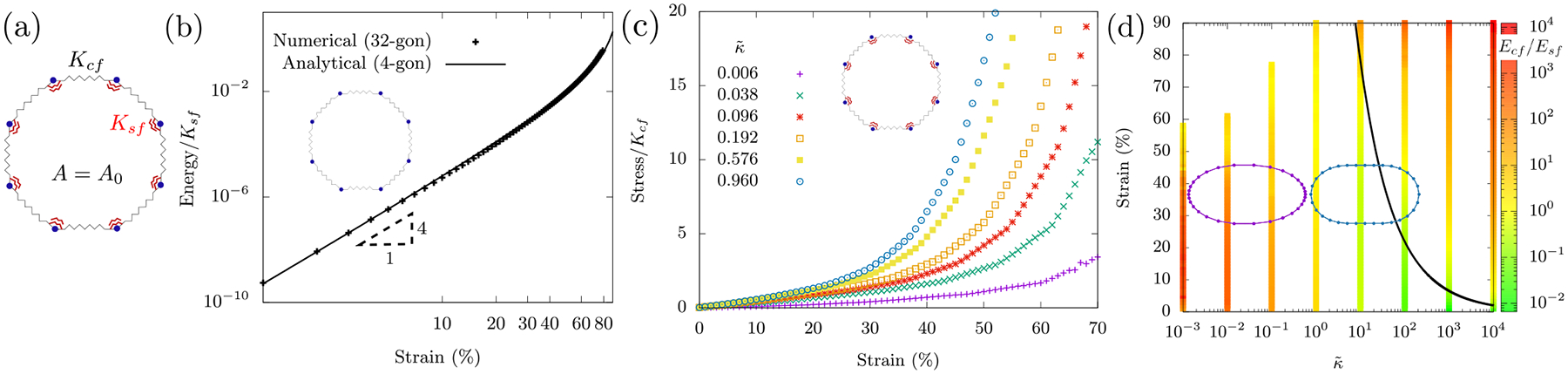
(a) The schematic of the system with central force spring (black) between neighboring vertices (blue) and angular spring (red) across a vertex. The spring constants are K_cf and K_sf respectively. The area of the polygon is preserved as the system is uniaxially compressed. (b) With just the central force springs, energy is seen to be quartic at small strain. Analytical calculations confirm the same (see Appendix (A)). (c) Adding angular springs to the system brings linear behaviour at small strain since bending energy is quadratic at small strain (see Appendix A). This delays the onset of non-linearity effected by the central force springs. The onset of non-linearity is tuned by changing $\tilde{k}$. (d) Heat map for the ratio of stretching and bending energy, E_cf/E_sf as a function of $\tilde{k}$ and strain. The solid black line is an analytical estimate separating the bending and stretching regimes. The shape of the polygon at 30% strain for $\tilde{k}=0.006$ (dark-violet) is ellipse-like and for $\tilde{k}=0.960$ (blue) is pill shaped. All numerical results were obtained using a 32-gon.