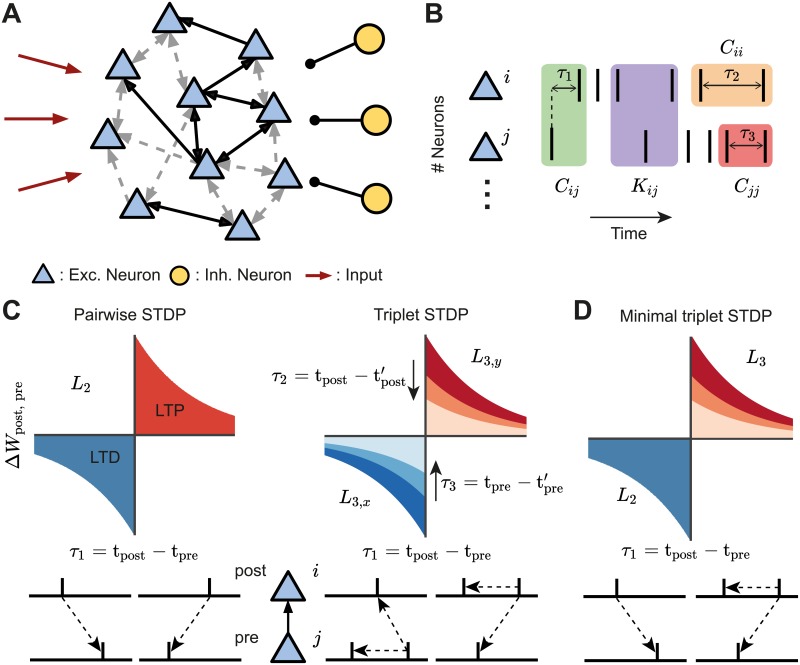
Fig 1. Framework set-up.

A. A network of excitatory neurons (light blue triangles) fire stochastically, while their activity is driven by unstructured external input (red arrows) and modulated by a population of inhibitory neurons (yellow circles). Excitatory connections among the neurons can be weak (gray dashed arrows) or strong (black solid arrows), unidirectional or bidirectional. B. Cumulants of the spike trains (see Eq 1). The second-order cumulants C_ij, C_ii and C_jj are calculated based on the time difference between a pair of spikes (cross-covariance in green; auto-covariances in orange/red). The third-order cumulant K_ij is calculated based on the time differences between three spikes (purple). The spike triplets can be two post- and one presynaptic spikes, or one post- and two presynaptic spikes. The time differences are: τ₁ between a presynaptic spike and a postsynaptic spike, τ₂ between different postsynaptic spikes and τ₃ between different presynaptic spikes. C. STDP-induced plasticity by pairs and triplets of spikes. Left: An example of a classical pair-based STDP rule, with a learning window denoted by L₂. Potentiation is triggered by a postsynaptic following a presynaptic spike (τ₁ = t_post − t_pre > 0), whereas if a presynaptic spike follows a postsynaptic spike (τ₁ = t_post − t_pre < 0), depression is induced. The total potentiation (depression) is given by the red (blue) area under the curve. Right: Examples of triplet STDP rules denoted by L_3,y and L_3,x. Potentiation (red) and depression (blue) are given by triplets of spikes: post-pre-post with a time difference ${\tau }_2={\text{t}}_{\text{post}}-{\text{t}}_{\text{post}}^{\prime }$, and pre-post-pre with a time difference ${\tau }_3={\text{t}}_{\text{pre}}-{\text{t}}_{\text{pre}}^{\prime }$, respectively. D. Minimal triplet STDP rule where potentiation depends on triplets of spikes L₃ and depression on pairs of spikes L₂.