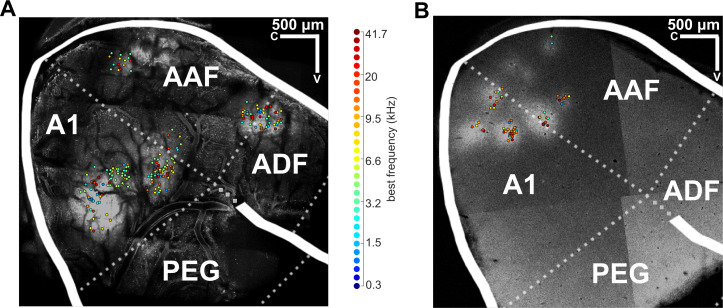
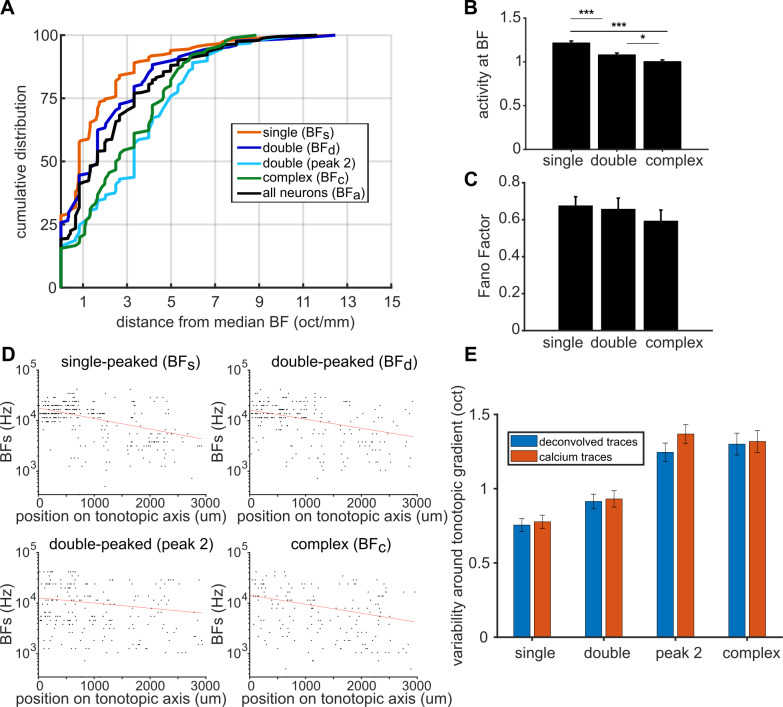
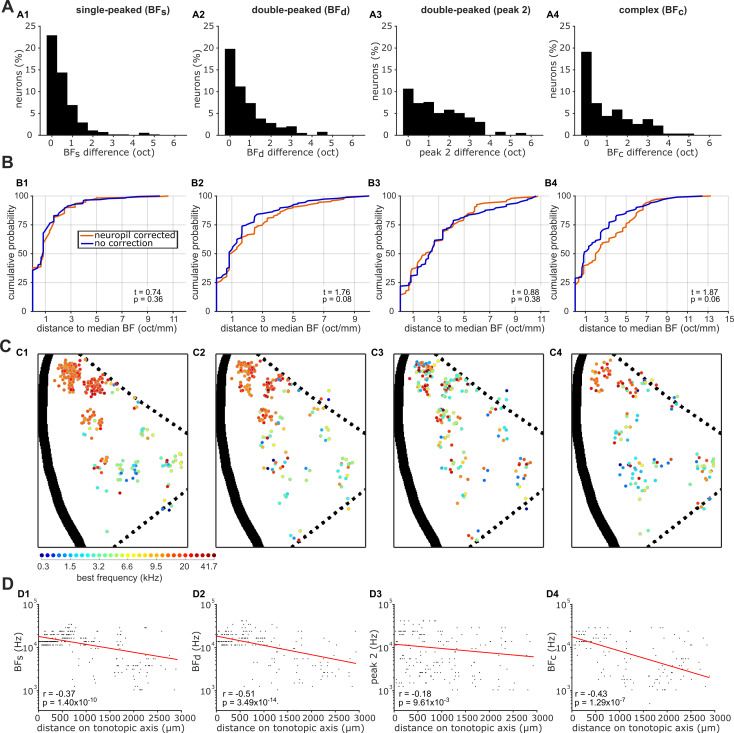
Figure 4. Global tonotopic organization of frequency preferences in ferret A1.

The anatomical locations of neurons imaged across eight ferrets were projected onto a template map of auditory cortex, shown in the top-left corner. Thick black solid lines indicate sulci, and black dotted lines indicate approximate borders between known cortical fields. The two boxes represent the location of A1 (large box; A) and the most dorsal region of A1 (small box; B). The colored line illustrates the tonotopic gradient. (A) The BFs of individual neurons are color-coded (legend above) and mapped onto A1. The spatial distributions of BF_s (A1), BF_d (A2), peak 2 of double-peaked neurons (A3), and BF_c (A4) are plotted separately. (B) Frequency preferences are mapped as in (A) for the dorsal tip of A1, where many neurons are occluded in (A). (C) BF_s (C1), BF_d (C2), peak 2 (C3), and BF_c (C4) of each neuron are plotted against the neuron’s position along the tonotopic axis on the template A1. Red lines show the best single-term exponential fits to the data, and Pearson’s correlations (r) with their p-values (p) are also shown.

Figure 4—figure supplement 1. Mapping data from individual ferrets onto a common template of auditory cortex.

(A) An image of the brain surface of ferret 3, acquired in vivo using two-photon calcium imaging. Individual frequency-sensitive neurons imaged across all fields are plotted on this map according to their anatomical position, where their color indicates their BF (color scale, right). The common template of auditory cortex is superimposed, where white solid lines indicate sulci and white dotted lines indicate approximate borders between known cortical fields (names in white text). The brain image and template were aligned according to the positions of sulci, and frequency responses of imaged neurons. Only neurons in A1 were included in our analyses. (B) A post mortem section of the auditory cortex of ferret 7, which was sliced parallel to the two-photon imaging plane (i.e. parallel to the surface of auditory cortex) and imaged with a confocal microscope. The BF of imaged neurons and the cortical template are superimposed, as in (A). A1 = primary auditory cortex; AAF = anterior auditory field; PEG = posterior ectosylvian gyrus (containing the posterior pseudosylvian and posterior suprasylvian fields); ADF = anterior dorsal field.

Figure 4—figure supplement 2. Mapping the peak-to-peak distances in double-peaked neurons.

(A), (B) Double-peaked neurons imaged across all ferrets are mapped onto the template A1, as in Figure 4A and B. Here, the color scale (below B) indicates the difference, in octaves, between BF_d (i.e. the frequency eliciting the strongest response) and peak 2 (i.e. the frequency eliciting the second-strongest response) for each neuron. (C) The octave difference between BF_d and peak 2 is plotted for each double-peaked neuron, as a function of its position along the tonotopic axis (as in Figure 4C). The red line represents the best single-term exponential fit to the data, and the coefficient (r) and p-value (p) of Pearson’s correlation are shown. (D) Distribution of the difference (in octaves) between BF_d and peak 2, across all double-peaked neurons. BF_d and peak two were on average 1.74 ± 0.07 (mean ± SEM) octaves apart, and their octave distances did not differ when BF_d was lower or higher than peak 2 (t-test: t = −0.17, p=0.87).

Figure 4—figure supplement 3. Comparison between deconvolved and non-deconvolved traces.

(A) Cumulative probability plots of the difference (in octaves) between each neuron’s BF and the median BF of all neurons in the same FRA class and imaging field. Distributions for different FRA classes are plotted separately: single-peaked BF_s; double-peaked BF_d; double-peaked peak 2; complex BF_c; and the BF_a of all three FRAs together. (B) Magnitude of the trial-averaged response at BF, calculated from non-deconvolved fluorescence traces. Responses were averaged across all neurons in each FRA class, pooled across imaging fields and ferrets (mean ± SEM). Significant results of t-tests are shown above the bars: * p < 0.05, *** p < 0.001. (C) Fano Factor values calculated at BF (mean ± SEM) for single-peaked, double-peaked, and complex neurons in non-deconvolved fluorescence traces. T-tests show no significant differences between groups (p > 0.05). (D) BF_s , BF_d, peak 2, and BF_c of each neuron are plotted against the neuron’s position along the tonotopic axis on the template A1. Red lines show the best single-term exponential fits to the data. (E) Average octave distance of each BF from the fit (red curves in D and Figure 4C) for deconvolved traces (blue) and non-deconvolved fluorescence traces (red) (mean ± SEM). T-tests show no significant differences between results of the two methods for each FRA type (p > 0.05).

Figure 4—figure supplement 4. Effects of neuropil contamination on local and global tonotopic organization.

(A) Distributions of the difference (in octaves) between BF_s (A1), BF_d (A2), peak 2 (A3) and BF_c (A4) calculated with and without neuropil signal subtraction. (B) Cumulative probability plots of the difference (in octaves) between each neuron’s BF and the median BF of all frequency sensitive neurons in the same imaging field. Distributions for BFs obtained with (red) and without (blue) neuropil correction are plotted separately. The results of t-tests between the two distributions are given in the bottom right of each plot. (C) Frequency preferences are mapped onto a template A1 as in Figure 4A, but here without neuropil correction. (D) Scatterplots show the frequency preferences of neurons as a function of their position along the tonotopic axis of the maps in C (as in Figure 4C), for neuropil-uncorrected data.